CONTRIBUTIONS OF A WING

As previously remarked, on airplanes with tails the wing contributions to the q deriv­atives are frequently negligible. However, if the wing is highly swept or of low aspect ratio, it may have significant values of C and Cmq and of course, on tailless air­planes, the wing supplies the major contribution. The q derivatives of wings alone are therefore of great engineering importance.

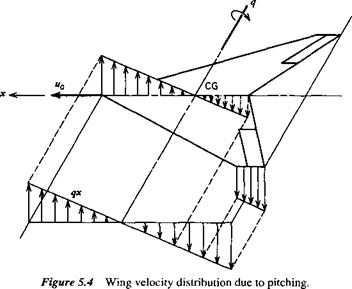
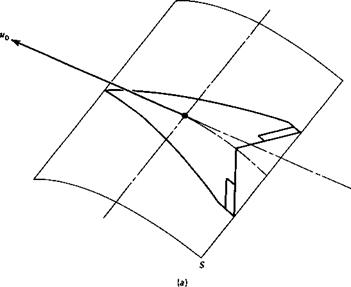
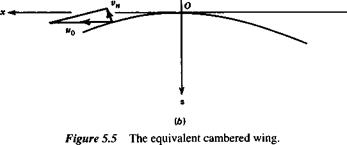
Unfortunately, no simple formulas can be given, because of the complicated de­pendence on the wing planform and the Mach number. However, the following dis­cussion of the physical aspects of the flow indicates how linearized wing theory can be applied to the problem. Consider a plane lifting surface, at zero ax, with forward speed u0 and angular velocity q about a spanwise axis (see Fig. 5.4). Each point in the wing has a velocity component, relative to the resting atmosphere, of qx normal to the surface. This velocity distribution is shown in the figure for the central and tip chords. Now there is an equivalent cambered wing that would have the identical dis­tribution of velocities normal to its surface when in rectilinear translation at speed n0. This is illustrated in Fig. 5.5a. The cross section of the curved surface S is shown in (b). The normal velocity distribution will be the same as in Fig. 5.4 if

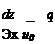
x

Hence

and the cross section of S is a parabolic arc. In linearized wing theory, both subsonic and supersonic, the boundary condition is the same for the original plane wing with rotation q and the equivalent curved wing in rectilinear flight. The problem of finding the q derivatives then is reduced to that of finding the pressure distribution over the equivalent cambered wing. Because of the form of (5.4,5), the pressures are propor­tional to qlu0. From the pressure distribution, CZq and Cmq can be calculated. The de­rivatives can in principle also be found by experiment, by testing a model of the equivalent wing.

The values obtained by this approach are quasistatic; i. e., they are steady-state values corresponding to ax = 0 and a small constant value of q. This implies that the flight path is a circle (as in Fig. 3.1), and hence that the vortex wake is not rectilinear. Now both the linearized theory and the wind-tunnel measurement apply to a straight wake, and to this extent are approximate. Since the values of the derivatives obtained are in the end applied to arbitrary flight paths, as in Fig. 5.2b, there is little point in correcting them for the curvature of the wake.

The error involved in the application of the quasistatic derivatives to unsteady flight is not as great as might be expected. It has been shown that, when the flight path is a sine wave, the quasistatic derivatives apply so long as the reduced frequency is small, that is,

k = — < (5.4,6)

2 u0

where to is the circular frequency of the pitching oscillation. If / is the wavelength of the flight path, then

c

к = тг —

so that the condition к 1 implies that the wavelength must be long compared to the chord, for example, l > 60c for к < 0.05.