Variation of velocity in vortex flow

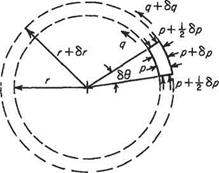
To confirm how the velocity outside a vortex core varies with distance from the centre consider an element in a thin shell of air (Fig. 5.12). Here, flow conditions depend only on the distance from the centre and are constant all round the vortex at any given radius. The small element, which subtends the angle 86 at the centre, is

Fig. 5.12 Motion of an element outside a vortex core circulating round the centre in steady motion under the influence of the force due to the radial pressure gradient.

Considering unit axial length, the inwards force due to the pressures is:

(p + 8p)(r + 6r)6Q-pr6Q – 2{p –]^8p)8r^86

which reduces to 8p(r — ^ 6r)66. Ignoring f Sr in comparison with r, this becomes r 8p 86. The volume of umt length of the element is r 8r 86 and therefore its mass is pr8r86. Its centripetal acceleration is (velocity)2/radius, and the force required to produce this acceleration is:

mass(ve!5dty£= rW

radius r

Equating this to the force produced by the pressure gradient leads to

r6p = pq26r since 66 Ф 0 (5.13)

Now, since the flow outside the vortex core is assumed to be inviscid, Bernoulli’s equation for incompressible flow can be used to give, in this case,

P + p<? = {p + 6p)+ іp(q + 6qf

Expanding the term in q + 8q, ignoring terms such as (6q)2 as small, and cancelling, leads to:

6p + pq 8q = 0 i. e.

6p = —pq6q (5-14)

Substituting this value for 6p in Eqn (5.13) gives

pq2 8r + pqrSq = 0 which when divided by pq becomes

q 8r + r 8q = 0

But the left-hand side of this equation is 6(qr). Thus

8{qr) = 0

qr = constant (5.15)

This shows that, in the inviscid flow round a vortex core, the velocity is inversely proportional to the radius (see also Section 3.3.2).

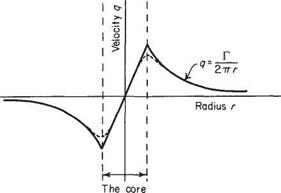
When the core is small, or assumed concentrated on a line axis, it is apparent from Eqn (5.15) that when r is small q can be very large. However, within the core the air behaves as though it were a solid cylinder and rotates at a uniform angular velocity. Figure 5.13 shows the variation of velocity with radius for a typical vortex.

The solid line represents the idealized case, but in reality the boundary is not so distinct, and the velocity peak is rounded off, after the style of the dotted lines.

Fig. 5.13 Velocity distribution in a real vortex with a core