BLASIUS FORMULA KUTTA-JOUKOWSK1 THEOREM

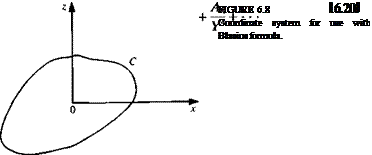
Consider the flow past a body whose contour is denoted by C (Fig. 6.8). Let the components of the aerodynamic force acting on the body be X and Z in the x and z directions, respectively. An integration of the pressure around the contour and an application of Bernoulli’s equation then leads to the Blasius formulas (see proof in Glauert,5 2 pp. 80-81):

X – iZ = ^ f [W(Y)]2 dY (6.19)

2 Jc

Let the free-stream velocity be Qvje~,a and let the circulation around C be Г (see Fig. 6.8). Then since the complex velocity is analytic outside of C, we can write W in a Laurent series about У = 0 (which is taken inside C)

Now substitute into the Blasius formula and with the use of the residue theorem we get

X-iZ = – ipQ„ Te~,a = pQSe-(-/2+л) (6.21)

or

X + iZ = pQSei(na+a) (6.21a)

The force is seen to act perpendicular to the stream Q* and has the magnitude D = 0 and L = pQJT- This result is called the Kutta-Joukowski theorem.