Essence of Fluid Mechanics

2.1 Introduction

Gases and liquids are generally termed fluids. Though the physical properties of gases and liquids are different, they are grouped under the same heading since both can be made to flow unlike a solid. Under dynamic conditions, the nature of the governing equations are the same for both gases and liquids. Hence, it is possible to treat them under the same heading, namely, fluid dynamics or fluid mechanics. However, certain substances known as viscoelastic materials behave like a liquid as well as a solid, depending on the rate of application of the force. Pitch and silicone putty are typical examples of viscoelastic material. If the force is applied suddenly, the viscoelastic material will behave like a solid, but with gradually applied pressure the material will flow like a liquid. The flow of such materials is not considered in this book. Similarly, non-Newtonian fluids, low-density flows, and two-phase flows such as gas liquid mixtures are also not considered in this book. The theory presented in this book is for well-behaved simple fluids such as air.

2.2 Properties of Fluids

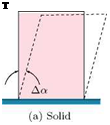
Fluid may be defined as a substance which will continue to change shape as long as there is a shear stress present, however small it may be. That is, the basic feature of a fluid is that it can flow, and this is the essence of any definition of it. Examine the effect of shear stress on a solid element and a fluid element, shown in Figure 2.1.

It is seen from this figure that the change in shape of the solid element is characterized by an angle Да, when subjected to a shear stress, whereas for the fluid element there is no such fixed Да, even for an infinitesimal shear stress. A continuous deformation persists as long as shearing stress is applied. The rate of deformation, however, is finite and is determined by the applied shear force and the fluid properties.

2.2.1 Pressure

Pressure may be defined as the force per unit area which acts normal to the surface of any object which is immersed in a fluid. For a fluid at rest, at any point the pressure is the same in all directions. The pressure in a stationary fluid varies only in the vertical direction, and is constant in any horizontal plane. That is, in stationary fluids the pressure increases linearly with depth. This linear pressure distribution is called hydrostatic pressure distribution. The hydrostatic pressure distribution is valid for moving fluids, provided there is no acceleration in the vertical direction. This distribution finds extensive application in manometry.

Theoretical Aerodynamics, First Edition. Ethirajan Rathakrishnan.

© 2013 John Wiley & Sons Singapore Pte. Ltd. Published 2013 by John Wiley & Sons Singapore Pte. Ltd.

Figure 2.1 Solid and fluid elements under shear stress.

When a fluid is in motion, the actual pressure exerted by the fluid in the direction normal to the flow is known as the static pressure. If there is an infinitely thin pressure transducer which can be placed in a flow field without disturbing the flow, and made to travel with the same speed as that of the flow then it will record the exact static pressure of the flow. From this stringent requirement of the probe for static pressure measurement, it can be inferred that exact measurement of static pressure is impossible. However, there are certain phenomena, such as “the static pressure at the edge of a boundary layer is impressed through the layer" which are made use of for the proper measurement of static pressure. The pressure which a fluid flow will experience if it is brought to rest, isentropically, is termed total pressure. The total pressure is also called impact pressure. The total and static pressures are used for computing flow velocity.

Since pressure is intensity of force, it has the dimensions:

Force _ MLT-2 Area L2

and is expressed in the units of newton per square meter (N/m2) or simply pascal (Pa). At standard sea level condition, the atmospheric pressure is 101325 Pa, which corresponds to 760 mm of mercury column height.