Synthesis of Chordwise Pressure

The ideas of synthesizing the distribution of chordwise pressure on the basis of contributions from camber and thickness envelopes are discussed by Abbott & von Doenhoff (1949). The velocity distribution about the airfoil, v/ V^, can be considered to be composed of three separate and independent contributions that are combined subsequently by linear superposition. These components of loading are: 1. A distribution of velocity vtfV00 cor­responding to that of the basic thickness form at zero AoA. 2. A distribution of velocity vc/ Voo corresponding to the camberline when operating at its ideal AoA. The ideal AoA corresponds to — «о, where «о is the AoA for zero-lift. 3. A distribution of chordwise load-

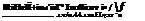
T c 1 глагііnгт р1пср1; олітаспапЛс tA tbp tV»in_oi Tfnil

11UJ IVUUUlg VIVJVl j VVU. VJ^/VUUJ tv VilV tllill Ull X VXi result for a flat-plate at AoA, although Abbott & von Doenhoff give tabulated values of Va/Voo for airfoils with finite thickness as obtained from other theoretical methods.

The local loading is equal to the difference in velocity between the upper and lower surfaces of the airfoil. In accordance with the principles of vortex sheets and thin-airfoil concepts, the velocity increment on one side of the airfoil surface is equal to the velocity decrement on the other surface. Therefore, the pressure coefficient can be obtained from

(7.35)

Values for vt and vc can be read directly from the tables in Abbott & von Doenhoff (1949). Values of vc will be tabulated for a specified design lift coefficient. The additional loading is a function of airfoil thickness, and is usually tabulated for a lift coefficient of 1.0. Because the results will usually be required for lift coefficients other than 1.0, they must be scaled by multiplying the values of the additional loading va by f(a) where

Да) = C’~Q|, (7.36)

C/o

and where Q. is the ideal lift coefficient, Q is the desired lift coefficient, and C/0 is the lift coefficient for which the values of va were tabulated.

The perturbation velocity distributions for a large number of camberlines and thickness envelopes are tabulated by Abbott & von Doenhoff (1949), and the method of superposition offers a simple and convenient way of constructing estimates of chordwise pressure for any derived airfoil shape. More importantly, however, the technique provides key elements for

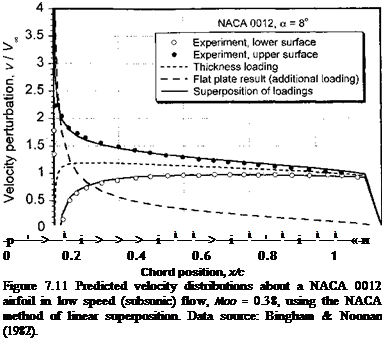the understanding of how airfoil shape affects the aerodynamic characteristics. An example of the technique is shown in Fig. 7.11, where the velocity distribution across the chord of a NACA 0012 airfoil is plotted in terms of the constituent parts. We see that the technique gives good agreement with the measurements. However, the technique is obviously restricted to low (subsonic) speeds and low angles of attack where the assumption of linearity can be justified (see also Question 7.4).

In the first instance, the effects of compressibility on the pressure distribution can be predicted using the Glauert rule, which gives the pressure distribution at any Mach number CPm in terms of the incompressible value CPi using

CPU = (7.37)

which is restricted to conditions below the critical Mach number where linearity in the airloads can be assumed.