The characteristics of a simple symmetric loading – elliptic distribution

In order to demonstrate the general method of obtaining the aerodynamic charac­teristics of a wing from its loading distribution the simplest load expression for symmetric flight is taken, that is a semi-ellipse. In addition, it will be found to be a good approximation to many (mathematically) more complicated distributions and is thus suitable for use as first predictions in performance estimates.

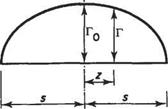
The spanwise variation in circulation is taken to be represented by a semi-ellipse having the span (2j) as major axis and the circulation at mid-span (Го) as the semi­minor axis (Fig. 5.30). From the general expression for an ellipse

or

(5.37)

This expression can now be substituted in Eqns (5.32), (5.34) and (5.36) to find the lift, downwash and vortex drag on the wing.

Fig. 5.30 Elliptic loading

Lift for elliptic distribution

From Eqn (5.34)

L = L, PVTil = //*T°V 1 “ ©2d*

i. e.

L = pVT 0тг-

whence

{pVirs)

or introducing

L = Cl-pV2S

giving the mid-span circulation in terms of the overall aerofoil lift coefficient and geometry.

Downwash for elliptic distribution

Here

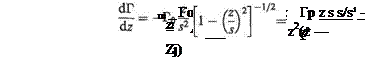
Substituting this in Eqn (5.32)

w*, =^[tt + zi/] (5.40)

Now as this is a symmetric flight case, the shed vorticity is the same from each side of the wing and the value of the downwash at some point z is identical to that at the corresponding point – z on the other wing.

So substituting for ±zi in Eqn (5.40) and equating:

^=ё[*+г,,1=й[’г■z,,1

This identity is satisfied only if / = 0, so that for any point z — z along the span

Го

4s

This important result shows that the downwash is constant along the span.

Induced drag (vortex drag) for elliptic distribution

From Eqn (5.36)

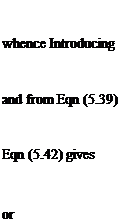^=/>“’rdz=/>Br»v/i-©5d2

— – = aspect ratio (Лі?)

Equation (5.43) establishes quantitatively how Cffy falls with a rise in (AR) and confirms the previous conjecture given above, Eqn (5.36), that at zero lift in sym­metric flight Сду is zero and the other condition that as (AR) increases (to infinity for two-dimensional flow) decreases (to zero).