EFFECT OF STRUCTURAL FLEXIBILITY

Many vehicles when flying near their maximum speed are subject to important aeroelastic phenomena. Broadly speaking, we may define these as the feedback effects upon the aerodynamic forces of changes in the shape of the airframe caused by the aerodynamic forces. No real structure is ideally rigid, and aircraft are no exception. Indeed the structures of flight vehicles are very flexible when compared with bridges, buildings, and earthbound machines. This flexibility is an inevitable characteristic of structures designed to be as light as possible. The aeroelastic phenomena which result may be subdivided under the headings static and dynamic. The static eases are those in which we have steady-state distortions associated with steady loads. Examples are aileron reversal, wing divergence, and the reduction of longitudinal stability. Dynamic cases include buffeting and flutter. In these the time dependence is an essential element. From the practical design point of view, the elastic behavior of the airplane affects all three of its basic characteristics: namely performance, stability, and structural integrity. This subject occupies a well-established position as a separate branch of aeronautical engineering. For further information the reader is referred to one of the books devoted to it (refs. 5.11 and 5.12).

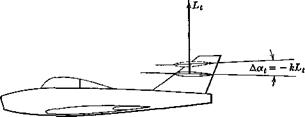
In this section we take up by way of example a relatively simple aero­elastic effect; namely, the influence of fuselage flexibility on longitudinal stiffness and control. Assume that the tail load Lt bends the fuselage so that the tail rotates through the angle Да, = —kLt (Fig. 7.9) while the wing angle of attack remains unaltered. The net angle of attack of the tail will then be

«■t = «■wb — e + h — kLt Fig. 7.9 Tail rotation due to fuselage bending.

and the tail lift coefficient at Se = 0 will be

CLt = = <*<(*«,6 -€ + »г – Щ)

But Lt = CLtyV>St, whence

0Lf = at(aab – e + it – kCLi§pV2St)

Solving for CLt, we get

1 + katStlpV*

Comparison of (7.4,2) with (6.3,26) shows that the tail effectiveness has been reduced by the factor 1/[1 + kat(p/2)F2(S’J. The main variable in this expression is V, and it is seen that the reduction is greatest at high speeds. From (6.3,36), we find that the reduction in tail effectiveness causes the neutral point to move forward. The shift is given by a dot) (7.4,3) where Д a, = a,(———————– 4 41 + kat±pV% J (7.4,4)

The elevator effectiveness is also reduced by the bending of the fuselage. For, if we consider the case when be is different from zero, then (7.4,1) becomes

CLt = аМ«,ь – e + it – hCL±pV*St) + «А

and (7.4,2) becomes

c = at(xWb ~ с + h) + aA

Ь 1 + katlpV%

Thus the same factor 1/(1 + katpV2St) which operates on the tail lift slope at also multiplies the elevator effectiveness ae.