Our heavyweight helicopter equal in the world does not have
In Rostov started production of the most load-lifting rotary-wing car The Russian holding «Helicopt[...]
EIGENVECTORS
The eigenvectors corresponding to the above modes are given in Table 6.4. They are for the nondimensionable variables, in polar form, the values given corresponding to n + і со. Eigenvectors are arbitrary to within a complex factor, so it is only the relative values of the state variables that are significant. We have therefore factored them to
|
|
|
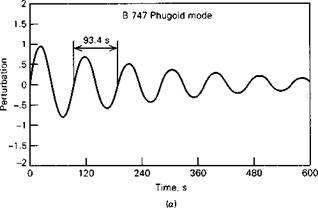
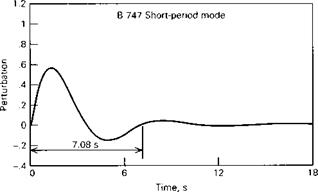
(6) Figure 6.2 Characteristic transients, (a) Phugoid mode, (b) Short-period (pitching) mode. |
 |
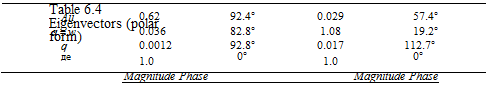make AO equal to unity, as displayed in the Argand diagram of Fig. 6.3. As we have chosen the positive value of o>, the diagrams can be imagined to be rotating counterclockwise and shrinking, with their projections on the real axis being the real values of the variables.
The phugoid is seen to be a motion in which the pitch rate q and the angle of attack change a are very small, but Ай and AO are present with significant magnitude. The speed leads Д 0 by about 90° in phase.
The short-period mode, by contrast, is one in which there is negligible speed variation, while the angle of attack oscillates with an amplitude and phase not much different from that of AO. This mode behaves like one with only two degrees of freedom, AO and a.
