FLUID DYNAMIC LIFT

Using the various components of longitudinal moment together with the downwash mechanism, it is now possi­ble to evaluate or to predict the static stability of plain wing-fuselage-tail configurations.

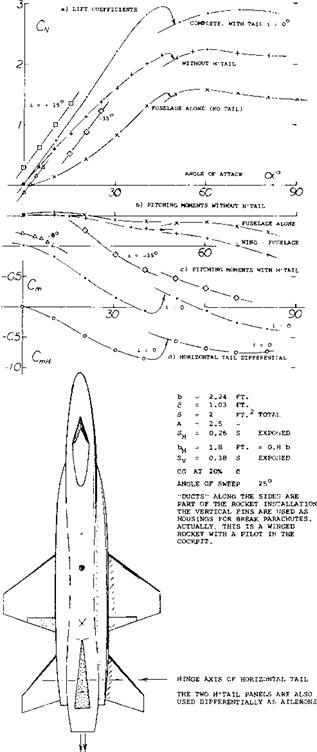
Moment Due to Tail Figure 15 presents characteristics of a simple airplane configuration. The stabilizing moment derivative (dC^dCY ) of the horizontal tail is found from the difference of the tests with tail on and tail off. The tail’s moment is theoretically

dCmJdCL= – (dCL /doc) H (SH/S)(^/c)(l +df/d(r)(do:/dCL)

(27)

In the example as in the illustration, the known terms in this equation are dCL/doc = 0.07, Su/S = 0.15,4 M/c = 3.4, so that VM = 0.5. By comparison of the stabilizing mo­ments, with and without the wing, we find (1 + (dt/doC)) = 0.45, so that dt/doc = — 0.55. The rest is as tested.

AVION W 5 WING: A = b/c = 6 RECTANGULAR AND STRAIGHT, dCj/daC = 0.078; 4(10) HORIZONTAL TAIL: SH/S – 0.14; ^/c =2.3; VH = 0.32; WITH CUT-OUT AERODYNAMIC 3ENTER AT x/c = 0.34, and z/c = 0.25, AS AGAINST WING LE CENTER OF GRAVITY ASSUMED AT x/c = 0.25, AND z/c = -0.40 REFERENCE (17,e) TESTED AT Rc = 4(10)5

IN THE GRAPH INDICATING:

A – AC^ = -0.09 CL DUE TO CG 9% AHEAD OF AC В – £Cm = -0.09 CL2 ШЕ TO CG 0.4 c BELOW AC C – <3Cm~ CL2 DUE TO VARIATION OF DOWNWASH df/dc<

Neutral Point. When moving the CG of an airplane aft stability reduces to zero when reaching the neutral point (neutral stability). For a given configuration, with a pitch­ing moment as in equation (27), this point is indicated by

(a/c)-(n/c) = dQn« /dCL (28)

To be stable, n must be longer than a (see figure 1). Since іц changes little when changing g and n, if the variation of the combined CL due to a change in CLW is small, the absolute value of the combined d/Cm/dCL (about the leading edge) is equal to n/c. The location “n” of the neutral point is thus aft of the leading edge. Usually, the fuselage contributes a positive Д (dCry/dCY), resulting in a forward shift of the AC while the horizontal tail provides the stabilizing dCniH/dCL, usually between — 0.1 and — 0.2, thus extending the neutral point, say to 30 or possi­bly 35% of the chord. All these variations are indicated by

Дх/с = – A(dC*/dCL) (29)

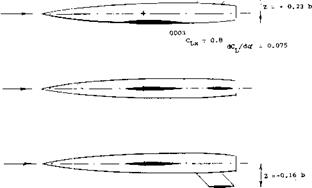
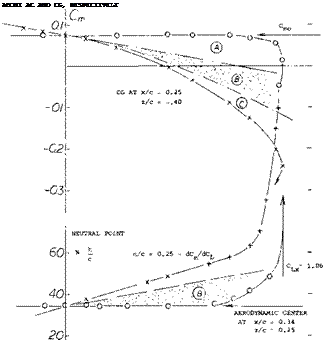
High Wing. Figure 24 gives an example for the neutral point of a “parasol” configuration. Between CL = 0 and 0.9, the neutral point moves from x/c = 0.34 to above 0.6. This variation is primarily caused by the stabilizing action of the wing’s lift at its AC in reference to the CG. In a manner similar to that in airfoil sections and/or in wings, an aerodynamic center can also be determined for an airplane configuration; the neutral point. This can ap­proximately by considering [105]

-2 C 02 04 Об 08 Ю CL

Figure 24. Longitudinal characteristics of a “parasol” type air­plane configuration. Aerodynamic center and neutral points, all including the horizontal tail, set at – 4.5 against wing zero lift line.

In the graph indicating:

A – С*, = ~ 0.09 CL due to CG 9%c ahead of AC В – Cm = – 0.09 CL2 due to CG 0.4 c below AC C – due to variation of downwash d/daf

where z = positive in a high-wing arrangement. Note that the influence of vertical displacement corresponds to that of a mechanical pendulum, the CG is suspended below the wing’s center of lift. It should also be noted that d(ACrr)/dCL = constant for a certain displacement “z” of the wing against the CG or vice versa. In the case of figure 24, it was found by trial and error, that the Cm(CL) function can be made into a line practically straight be­tween Cl = 0.2 and + 0.8, when raising the reference point to the level of z = 0.24 c, above the wing’s гею lift line. The neutral point NP (aerodynamic center of the configuration, including the horizontal tail) is thus found to be at

x = 0.34 c; and z = 0.24 c

measured from wing leading edge and above its zero lift line (which corresponds to oc0 = — 0.7 ). The explanation for the comparatively high location of the NP (in figure 24) may be found:

(a) in lift (and drag) originating and/or increasing in the rear end of the low aspect ratio “square” fuselage, as the angle of attack is increased;

(b) possibly of some progressive viscous interference of the fuselage with the wing’s downwash;

(c) in the low position of the horizontal tail in relation to the wing and its downwash sheet (see later under “low horizontal tail”).

It is thus seen that the downwash and its variation, as the tail moves up or down as a function of CL, contribute to the position of the “aerodynamic^center.” In view of the reduction of stability due to propeller and slipstream action (discussed later) an increase of dCrrJdCL as indi­cated in equation 30 may be desirable to a degree. On the other hand, strong stability at high lift coefficients, makes control of the airplane during a landing maneuver diffi­cult, particularly when the airplane is designed for tail – down and tail-first type of contact with the ground

Wing Wake. As shown in (15,d) the turbulent viscous wake emanating from the trailing edge of an average plain wing section, has the following characteristics at a con­ventional location of the horizontal tail:

x = 2 c, measured from trailing edge of wing

0/c = 0.5 Cds~0.005 = momentum thickness ratio

6л ~ 1.10 = displacement thickness of wake Vmin ~ 0-94 V = minimum speed, in center of wake S ^ 0.1 f c = total thickness of wake [106]

WING: 0018/09, 0.5 TAPERED A = 6, Rf = 8(10)6 VET deader.= 0.080 (W’OUT TAIL), = 0.086 (WITH TAIL) FUSELAGE: ROUND, 1/d = 5.9; 1/b = 2/3 HORIZONTAL TAIL SURFACE: S^S = 0.18; lH/c = 2.6 0009 ELLIPTICAL, AH = 4.5 HORIZONTAL TAIL CONTRIBUTIONS:

		(WdX	dc/dd		d</dcL2
HW	-3.16	+0.16	-0.47	0.53	-0.8°	+ 1°
MW	-3.15	+0.15	-0.50	0.50	-0.6°	0
LW	-9.14	+0.14	-0.53	0.47	ZERO	-1°

A INDICATES FUSELAGE VISCOUS LIFT EFFECT В REPRESENT A PLUS DOWNWASH VARIATION

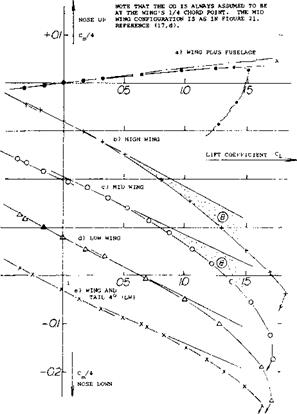
Figure 25. Influence of vertical location of the horizontal tail surface as against wing and vortex sheet. Note that the CG is always assumed to be at the wing’s 1/4 chord point. The Mid Wing configuration is as in figure 21. Reference (17,d).

Depending upon the geometrical arrangement, the wake sheet may meet the horizontal tail surface, at a certain angle of attack or lift coefficient. A theoretical and ex­perimental study (15,a) indicates that in this event, the lift of the tail surface basically corresponds to the reduced dynamic pressure within the wake. However, within a field of flow with a velocity gradient in the direction normal to its span, an airfoil becomes attracted toward
the side of increasing velocity. The result can be a certain irregularity in the forces or moments supplied by the horizontal tail surface. Pitching moment coefficients of a simple airplane configuration are plotted in figure 25:

(a) for wing plus fuselage, mid-wing configuration,

(b) with wing and CG in high position

(c) for a symmetrical mid-wing combination,

(d) for a wing and CG in lowered position,

(e) for a wing and tail setting of + 4° against the fuselage.

By reasons of symmetry, configuration (b) must have an inflection around C L and/or oc = zero. There are evi­dently “dents” in the C^CjJ functions of (d) and (e), at CL ~ 0.3 and 0.4, respectively. At these critical lift coefficients, the slope dCm/dCL is locally reduced. Of course, in the operation of an airplane, the “mixing” action of the propeller (if any) or variations of wing shape causing a distortion of the vortex sheet (such as dihedral, cut-outs, flaps) may render the dent in the func­

tion insignificant.

Low Horizontal Tail From the date on the mid-wing configuration in figure 25, it is seen that the Cm(CL) function is curved in such a manner that the value of dCm/dCb increases with lift coefficient. The reason for this is the wing plus fuselage alone function of VSCL, figure 25, and evidently the fact that the tail (when below the vortex sheet) moves into the field of reducing down – wash shown in figure 20. At a conventional tail location x/b ~ 0.6, the variation may be approximated by equa­tion (26). For the mid wing in figure 25, the angular displacement of the horizontal tail away from the vortex sheet is ^ — 0.25 oc, so that Az/b = – 0.25 (doc/dCL) CL ^ – 0.06 CL, at x/b = 0.6. The resultant increment of the angle of attack at the tail corresponds to A ((fc/doc)* – (A z/b) d£x/doc = -1.5 (0.06) C L0.5

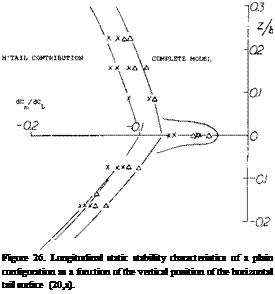
Vertical Position of Tail There is considerable evidence indicating that an improvement of stability does take place, as the horizontal tail moves below the vortex and wake sheet. For example, the midwing configuration in figure 25 shows an increment of the tail-moment con­tribution at CL = 1, in the order of 8% (not counting the increment due to fuselage). The high wing or low-tail arrangement m the same graph has a tail moment of 12%.

b = 11 INCH

Another advantage of the latter configuration (with the CG at the wing’s quarter point) is the fact that the horizontal tail is always below the vortex sheet where (as in figure 20) the downwash is less than the maximum. As a consequence, the low-tail configuration exhibits a dCm/dCL = 0.18 (at CL = 0.8), while the mid-wing type has only — 0.16. Results of a systematic investigation (20,a) are plotted in figure 26 and indicate:

(a) With the horizontal tail either above or below the vortex sheet, the stabilizing contribution of the tail is increased in comparison to that on fuselage center line.

(b) The improvement is greater below than above the wing chord. The derivative d£/dz is evidently favorable be­low, and unfavorable above the vortex sheet.

(c) Directly on the fuselage (at its center line) the tail contribution is extremely low. It must be assumed that viscous interference (described in the “horizontal tail” section) is maximum here.

‘T” Tail. An extreme measure to avoid interference with the downwash sheet as well as with any wake, is to place the horizontal tail as high as possible. An example of a very high location of the horizontal tail surface, on top of the vertical surface (thus forming a “T” tail) is shown in figure 27. The tested pitching-moment derivatives (at lift coefficients below ~ 0.5) are as follows:
where do^ц /doC = (15 — 11.5)/15 = 0.23 only. Using the ratios as indicated in the illustration, and the lift angle of the wing doc /dCL = 15 as tested, the value dCmH/dCL~

— 0.085 is obtained. This compares with the test value of

– .08 shown on figure 27. Considering now the horizontal

WING: 0.6 TAPERED A = 4, 65A008 H’TAIL: SH/S = 0.2; j^/c = 1.8 h/ H = 0.6 FOR "T" TAIL

HORIZONTAL TAIL CONTRIBUTION:

dCmH/dCL Лх/5 ON FUSELAGE CL -0.08 +8%

MT" TAIL POSITION -0.25 +25%

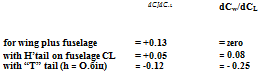where the members in the last column indicate the stabi­lizing differentials of the horizontal tail. The + 0.13 is essentially due to the fuselage, hinged at 0.54 of its length (a point not typical in conventional airplanes). With the horizontal tail located on the fuselage center line, the configuration is not stable. Analysis using equations 22 and 23 suggests that the downwash angle is

de/dCL ~ — 9.2 — 2.3 = — 11.5°

which is 2.5 times the induced angle of attack. The influence of the fuselage upon the effective aspect ratio is estimated to result in AL = 0.7 (4) = 2.8. Using the corresponding lift angle of

(doc/dCL)M = 10.5 + 6.5= 17°

the contribution of the horizontal tail then is calculated to be

^mn/dCL – — (doCM /d<X ) (SJS) (4 /с) (da: /dCL )/(doC /dCL )Figure 27. Influence of vertical position of the horizontal tail

(32) surface (20,d) on longitudinal stability. Rc = 8(10)6.

surface raised to the top of the vertical tail, we may expect that the downwash is reduced to 0.6 of that assumed above (see figure 20). Fuselage interference is assumed to be zero; in fact, the upper side of the hori­zontal surface is completely undisturbed. Since AH = 4 = A as in the wing, we obtain dotCM/dc*r = (15 — 7)/15 = 0.53, which is more than twice the 0.23 ratio for docH/dcxT above. Consequently:

dCmw/dCL = – 0.53 (0.2) 2.1 = – 0.22

while – 0.25 was obtained from test (see figure 27). The “T” tail configuration thus does two things:

it avoids the downwash maximum near the vortex sheet,

it avoids viscosity-induced fuselage (wake) interference.

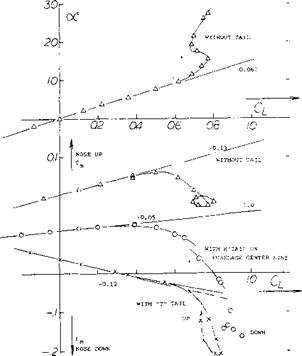
“71” Tail at High Angles: While the “T” tail, as in figure 27, is safely placed outside (above) the wing wake, the variation of downwash as in figure 20, should be expected to reduce stability, in the range of higher lift coefficients corresponding to what is explained above under “low horizontal tail”. Indeed, comparison of the C^Cl) func­tions in figure 27, without tail and with “T” tail, reveals a reduction of the stabilizing tail moment between CL = 0.3 and 0.6, similar in absolute magnitude to the stabilizing amount of I 0.45 I, derived above for a low-tail con­figuration. Therefore, the advantage of the “T” tail ar­rangement rests primarily in the elimination of fuselage interference. Disregarding structural considerations, a really high location of the horizontal tail has another disadvantage. At very high angfes of attack, the horizontal surface is bound to get into the wake from the stalled wing. As seen in figure 27, the pitching moment then turns unstable (into the positive direction) thus presenting a situation which is likely to get into the “deep stall condition, Chapter XVI.

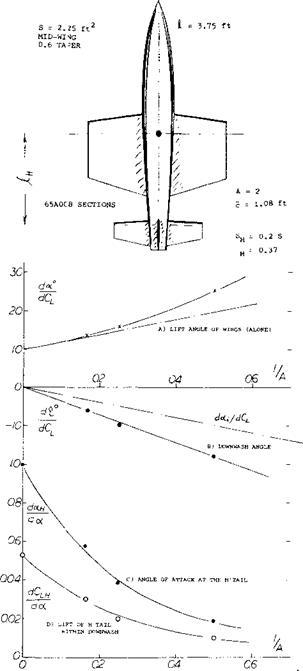
Aspect Ratio. A given tail plus fuselage assembly was tested (17,f) in combination with 3 wings having the same area, but differing in aspect ratio, between 2 and 6. The lift of the horizontal tail is found as the differential between the complete configuration and wing plus fuse­lage (tested without tail). Including for A = go, or 1/A = 0, the slope (dCL/do^ )й of tail plus fuselage tested alone, figure 28 demonstrates how the lift of the tail is reduced with a reduction of aspect ratio on account of increased downwash. It follows that

doCH/da: = (dCLH/doe)/(dCL/da: ) (33)

and del doc = doc jdoc – 1, so that

de/dCL = (docH/doc)(doc /dCL) – doc /dCL

where doc/dCL = lift angle of the respective wings as tested (plotted at the top of the graph). It is thus seen

that down wash increases and the stabilizing contribution of the tail reduces as the aspect ratio of the wing is reduced. For example, at A = 2, where ijc = 0.37 //1.08 = 1.3 and V =1.3 (0.2) = 0.26, the tail contribution is dCmw/dCL = – (dCLH/doO Vw (d(X/dCL) = – 0.03 (0.26) 25 = — 6.5%, which is only ~ 1/3 of what it is in average conventional airplane configurations.

Figure 29. Longitudinal characteristics of a model (22,b) similar to the NASA and North American “X-15”, tunnel-tested at low speeds (Rc = 2(10)fe).

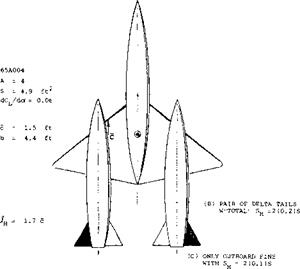
Big Fuselages. Small aspect ratios are used on winged missiles (21), where not only down wash is a problem but also the size of the body in comparison to wing and tail is critical. Another example involving small wings and a large fuselage, is the high-altitude research craft X-15. Charac­teristics of a model similar to this airplane are presented in figure 29. The maximum width of the body is 0.34 b; its plan-form area is — 1A times total wing area. Analysis of these data show:

(a) The lift curve slope (without horizontal tail) within the range of small angles of attack, dCL/doC = 0.055, corresponds to A = 2.5.

(b) The maximum lift coefficient (without tail) is CL~ 0.7 CM= 1.5, at oL =45°.

(c) When adding the horizontal tail, its contributions to lift and pitching moment are not linear.

(d) There is a critical angle of attack near 50 , where forces and moments exhibit discontinuities.

The non-linearities (c) and the discontinuities seem to be a consequence of the fuselage flow pattern (separation). It must be noted, however, that the wing eliminates most of the fuselage lift, by way of downwash, so that the lift- curve slope as in (a), is fairly constant.

Tail Contributions. The interaction between fuselage and horizontal tail in the configuration as in figure 29, is complex. A qualitative analysis is as follows:

(a) By comparison of dC^/di = — 0.026 with dCL/di = 0.020, a tail length = (0.027/0.020)c = 1.35 c is found.

(b) Based on exposed area of S ц = 0.26 S, the lift-curve slope of the tail is dCLH/di = 0.020/0.26 = 0.077, which seems to be proper for an “exposed” aspect ratio of A = Ъгц /S ц = 6.3.

(16) Simple aiqilane configurations in wind tunnels:

a) Schlichting, Interference, Ybk D Lufo 1943, Rpt IA028; see (10,e).

b) Gruenling, Investigation of Me-109 Model, ZWB UM 7857 (1944).

d) Sherman, 17 Combinations, NACA T Rpt 678 (1939).

e) Service Technique Aeronautique (Belgium), Bull 15, Feb 1935.

(17) f) Stivers, NACA TN 4238 (1958) and NASA D-13

(1959) .

g) Fournier, Three-Body Configuration, NASA TN D-217

(1960) .

(18) Influence of vertical CG location:

a) Andrews, Flight (Aircraft Eng) 1937 No. 1474 & 1479.

b) Francis, Notes on Stability, ARC RM 1833 (1938).

c) Bryant, “Puss Moth” High Wing, ARC RM 1687 (1935).

(19) Further variants of the configurations as in figures 25,26,27, references (17,f)(18,d)(20,d), are reported in NACA RM A52D23/E01/L15a, in TN 3551,3857, and in NASA D-13; between 1952 and 1959.

(c) At <X = 20° , the tail moment differential is A С mul A <X = — 0.022, in presence of the wing. This is less than the dCm/di = — 0.026 above.

(d) Again at oc = 20°, the lift differential due to tail is ACl/A<X – 0.016. Combining this value with the derivative in (c), a tail moment arm Лц = (0.022/0.016)c = 1.38 c is obtained.

The difference between the derivatives as agains: j and <x, is due to downwash as well as to a more or less unknown contribution of the fuselage. On the basis ofS*» (total) =

2.1 S ц (exposed) = 0.55 S, a (AC L/Да: ) и ~ 0.016/0.55 = 0.029 is obtained. Comparison with 0.077 as in (b) leads to doc н I doc = 0.029/0.077 = 0.38 and df/doc = – 0.62. These values seem to be reasonable. More accurate pre­dictions cannot be made, however, for a configuration:

where the fuselage is long and wide,
where the tail length is no longer than ^ wing chord.

To show the problematic character of the analysis, the downwash as derived above, is only 1.4 times the induced angle, which seems low.

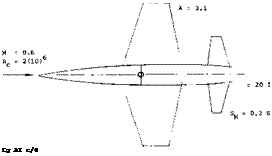
X-15. The model in figure 29, is evidently one repre­senting the NASA-North American research airplane “X-15”. Flight-test reports (22,c) reveal that the airplane is flown approximately:

Long Fuselage. The configuration in figure 30 serves first as another illustration for a long fuselage (forward). As­suming that the body in the middle may have a weight equal to 1/4 of the total, its addition moves forward the combined CG more than 20% of the “mac”; thus A (dCrr/dC _ ) ~ — 0.20, on account of fuselage weight. Its aerodynamic contribution is, on the other hand, Д (dCm/dCu ) = + 0.07. A long fuselage forward thus more than cancels its destabilizing effect; and we have an ex­planation, how long-nosed configurations such as that in figure 29, for example, can be stable in longitudinal direc­tion.

Zero-Lift Moment. Theoretically the fuselage does not develop lift. Its free moment (+ 0.07) has to be com­pensated, however, by a positive lift force in the hori­zontal tail. At higher lift coefficients, say at C L = 1.0, therefore, АСтн ~ — 0.07, which means in the con­figuration considered a AClh = + 0.07/0.66 ~ 0.1 and a total CLH ~ 0.2. This would not be a problem; in fact, in an airplane using wing flaps, the fuselage moment would tend to overcome the negative (nose-down) moment pro­duced by those flaps. On the other hand for a range of small lift coefficients (at high speeds) near Cb = 0, the differential depends entirely upon the angle “i” at which the wing is set against the fuselage. In a convention design, і (measured at the wing’s zero-lift line) is in the order of

at c< = 10° and with і = – 12° launching

at а: = 5° and with і = – 9° climbing

at oc = 15 ° and with і = – 20° recovering

s

This means that operation is at C L between 0.2 and 0.8. This range is small in comparison to that in figure 29. Other data and expected performance (22) of this airplane are:

b =	22 ft	T* =	60,000 lb	W – 31,000	lb with fuel
M =	^ 6 ~		3,500 kts	W = 13,000	lb burnt-out
l =	50 ft	s =	200 ft2	max altitude	~250,000 ft

(20) Influence of vertical location of the H4a. il:

a) Stivers, Vertical Location of Tail, NACA RM A57I10.

b) Multhopp, “T” Tails, Aero Digest, May 1955 .

c) Interaction with V’tail, see NACA TN 1050 & 2907.

d) Letko, in connection with (17,c), NACA TN 3481 (1955).

(21) Nielsen, Wing Body Tail Combinations, NACA T Rpt 1307 (1957).

(22) Aerodynamic characteristics of the X-15 research airplane:

a) Clark, Space Flight, Aero Space Engg, April 1959 p 43.

b) Bowman, Tunnel-Tested Characteristics, NASA TN D-403 (1960).

c) Flight Test Analyses, NASA TN D-615,623,723,1057,1059,1060,1159 (1960/62).

d) Weil, Review of Operation, NASA TN D-1278 (1962).

f) For data, see Jane’s All the World’s Aircraft 1961/62 p 292.

Figure 30. Three-body airplane configuration tested (17,g) at M = 0.2 and Rc := 2(10)4*. See text for principle results.

several degrees, so that the fuselage may be in horizontal position when cruising. Thus і = 0.3/0.06 = 5 , for ex­ample, for an assumed dCL/d<Y = 0.06. To balance the fuselage moment at CL =0, for instance high speed dive, a negative (down) load in the horizontal tail is required. In the example considered, that load would be in the order of 50% of the airplane’s weight (estimated on the basis of conventional values for tail volume et cetera), assuming a terminal speed two times the cruising speed and disre­garding any changes due to compressibility.

Taper Ratio. It is explained in the “downwash” section, that rectangular wings have less, and tapered plan forms more downwash in their center plane. Using a final (rolled-up) ratio t/ocL = 2.6 and an increment of at least 0.1 (as derived from equation 23),

d£/doc = – (tlocL )(d(*L /doc) = – 2.7 (5/17) = 0.8

or even higher, can be estimated for the doubly-triangular wing shape in figure 30. Analysis of the wide and large tail surface (S ц/S = 0.4) stretched out between the two nacelles (with A H = 4, plus end-plate effect supplied by the vertical fins) leads to an average effective ratio o^h /ос somewhat below 0.2. The configuration shown, or any similar, highly tapered wing, is thus rather ineffi­cient in regard to longitudinal stability.