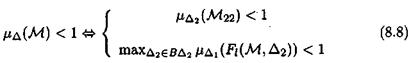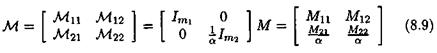A SECOND і/ UPPER BOUND

An alternative mixed v upper bound is proposed in this section (Fer­reres and Fromion, 1999). The approach, which consists in transforming the v problem into an augmented p problem, basically uses the Main Loop Theorem.

2.1 CHECKING A SMALL GAIN CONDITION DESPITE MODEL UNCERTAINTIES IS A SKEWED n PROBLEM

• First reshape the standard interconnection structure of Figure 8.1.a (with A = diag(A{, A2)j into the structure of Figure 8.1.b and partition M compatibly with the Aj’s as:

Remember finally in Figure 8.1.b that the LFT transfer Fi(M, Д2) between w and z (with Ді = 0) can be computed as:

Fi(M, Д2) = Mn + Ml2A2(I – M22A2)~lM2l (8.6)

Ді is to be maintained inside its unit ball, while the size of Д2Іs free.

Д2 is moreover supposed to contain only real (repeated) scalars.

• Lemma 2..1 is essentially a skewed version of the Main Loop Theorem (see subsection 4.4 of chapter 1).

LEMMA 2.

Proof of Lemma 2..1: the Lemma is essentially a scaled version of the Main Loop Theorem:

with:

kmax — ШШ шє[0,оо]

The rest of this subsection considers the case of a full complex block Ді: the general case of a mixed model perturbation Ді will be considered in the following subsection. The following Lemma is essentially a restate­ment of Lemma 2..1, since цаі = о in the case of a full complex block

Ді-

LEMMA 2..2 If v(M) < a, then ^а2(^22) < and:

max a(Fi{M, Д2)) < 1 (8-11)

A2£^BA 2

Checking a small gain condition despite model uncertainties Д 2 is thus a skewed ц problem. It suffices indeed to compute an upper bound of v(M) so as to compute an upper bound of й(М):

v(M) = 1 /тіп(к / ЗД2 Є кВД2 with a(Fi(M, A2)) > 1)

іщу represents the maximal size of the model uncertainty Д2, for which the small gain condition Д Д ( Д, Д 2 ) ) < 1 is satisfied.