Our heavyweight helicopter equal in the world does not have
In Rostov started production of the most load-lifting rotary-wing car The Russian holding «Helicopt[...]
Pitching Oscillations
For harmonic pitch oscillations, additional terms involving pitch rate a appear in the equations for the aerodynamic response. The forcing is now given by a = aelcot, and the pitch rate by a — iwotelwt. In this case, the lift coefficient is
 |
 |
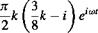
(8.32)
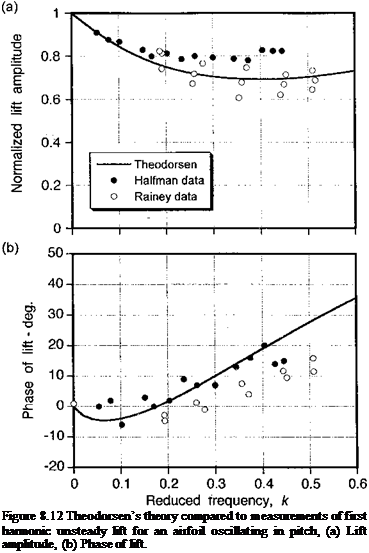
The results from these predictions are compared with experimental measurements in Figs. 8.12 and 8.13, again in terms of the normalized lift and pitching moment amplitude about the 1 /4-chord (using Eq. 8.28) and their corresponding phase angles versus reduced frequency. In addition to the low Mach number results of Halfman (1951), this figure shows data measured by Rainey (1957), which are for a Mach number of about 0.3 and a Reynolds number of 5.3 x 106. It is significant in the latter case that a reduced frequency of up to 0.6 was obtained in the experiment, which gives a good opportunity to examine the validity of the linearized incompressible aerodynamic theory at higher reduced frequencies. As shown in Fig. 8.12, the lift amplitude initially decreases with increasing к because of the effects of the shed wake. The amplitude’begins to increase again for к > 0.5 as the apparent mass
|
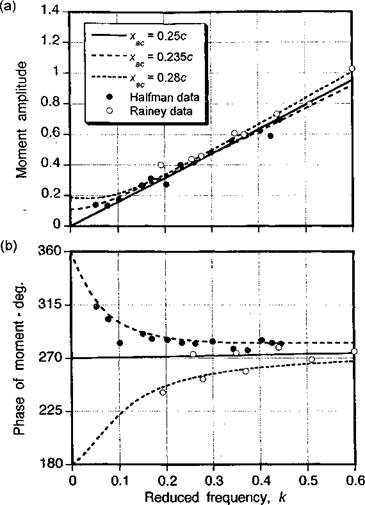
Figure 8.13 Theodorsen’s theory compared to measurements of first harmonic unsteady pitching moment for an airfoil oscillating in pitch, (a) Pitching moment amplitude about 1/4-chord, (b) Phase of pitching moment. |
forces begin to dominate the airloads. This is also shown by the phase angle, which exhibits an increasing lead for к > 0.3. For the lift amplitude, Theodorsen’s theory compares well with Rainey’s results. The agreement with Halfman’s results are not quite as good for the lift amplitude but are better in phase. In Fig. 8.13 it is apparent that the amplitude of the 1/4-chord pitching moment increases quickly with increasing k, with the agreement of theory and experiment being excellent. Again, the phase of the pitching moment response shows a behavior that can be explained in terms of shift of the aerodynamic center from the 1 /4-chord. As for the plunging case, Halfman’s data suggest an aerodynamic center at 23.5% chord. Rainey’s data, however, suggest that the aerodynamic center is further back at 28% chord. Overall, the correlation obtained between Theordorsen’s theory and experimental measurements for airfoils oscillating in pitch and plunge is quite good, giving considerable support to the validity of Theodorsen’s theory, at least for low Mach numbers and up to moderate values of reduced frequency.
