Case Study: Wall Temperature and Skin Friction at the SANGER Forebody

We discuss the results of Navier-Stokes/RANS solutions ([41], based on [42, 43]) for the forebody of the lower stage of the SANGER TSTO space transportation system, Section 1.1, which is a CAV. The numerical simula­tions were made for a flight situation and a wind-tunnel situation (H2K is a hypersonic wind tunnel of DLR at Koln-Porz, Germany), Table 7.9. We check and interpret the computed data with the help of the approximate, reference-temperature extended boundary-layer relations for flat surface por­tions which we have presented in the preceding sub-sections.

Table 7.9. Parameters of the SANGER forebody computation cases. L is the length of the forebody, see Fig. 7.11. Case Moo H [km] Too [K] T„ [K] Relo [1/m] L [m] а П Flight situation 6.8 33 231.5 radiation cooled variable 1.5-106 55 6 H2K situation 6.8 – 61 300 8.7-106 0.344 6

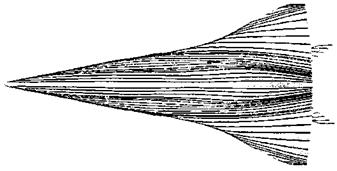
In Figs. 7.8, [44], and 7.9, [45], the configuration is shown with computed skin-friction line patterns for the case under consideration. The first ramp of the inlet—not indicated in Fig. 7.8—lies at approximately 67 per cent length at the flat lower side. There the skin-friction line pattern indicates a flow between the primary attachment lines (not visible) which is to a good approximation parallel. This assures an effective pre-compression as well as the desired two-dimensionality of the inlet onset flow [36].

The presented and discussed results are the wall temperatures and the skin-friction coefficients at the lower, and partly at the upper symmetry line of the forebody. For the flight situation several assumptions were made re­garding surface-radiation cooling, gas model, and state of the boundary layer (laminar or turbulent).

We consider first the results for the lower (windward) symmetry line of the forebody. We see in Fig. 7.10 that the state of the boundary layer, laminar or turbulent, does not affect strongly the adiabatic (recovery) wall temperature Tw = Tr (cases e = 0). This can be understood by looking at the definition of the wall-heat flux in eq. (7.156). If the temperature gradient at the wall is zero by definition, the thermal conductivity к at the wall (always the laminar value), and in its vicinity (laminar or turbulent) can have any value with­out strong influence on the balance of thermal convection and conduction, compression and dissipation work, Sub-Section 4.3.2. The zero temperature gradient is also the reason why Tr does not depend on the inverse of some

Fig. 7.8. The lower stage of the SANGER TSTO space transportation system seen from below [44]. Computed pattern of skin-friction lines at the lower side without the airbreathing propulsion system. The free-stream comes from the left.

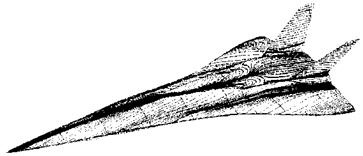
Fig. 7.9. The lower stage of the SANGER TSTO space transportation system— without the upper stage—seen from above [45]. Computed pattern of the skin – friction lines at the upper side without the upper stage. The free-stream comes from the left.

power of the boundary-layer running length x, like the radiation-adiabatic temperature Tra or qgw.

Another explanation for the small influence of the state of the boundary layer can be obtained from a look at the relation for the estimation of the recovery temperature Tr at flat plates, eq. (3.7). If the recovery factor r is equal to VPr for laminar flow, and equal to /Pr for turbulent flow, Tr will not be much different for laminar and turbulent flow, because for air Pr = 0(1). The recovery temperature estimated with eq. (3.7) is constant. Note, however, that the computed Tw drops from the nose region, perfect gas Tw « 2,300 K (total temperature: Tt = 2,372.4 K, recovery temperature laminar with eq. (3.7): Tr = 2,069.4 K), by about 150 K, and only then is approximately constant.28

The flow past the lower side of the forebody is not exactly two-dimensional, Fig. 7.8. Hence all results are only more or less monotonic in x/L.

2500

Tw [K]

2000

e= 0 e« 0 o c – 0 c – 0.85 E – 0.85

1500 –

1000

500 –

Fig. 7.10. Wall temperatures along the lower and the upper symmetry line of the forebody of the lower stage of SANGER in the flight and the wind-tunnel (H2K) situation [41, 42] (configuration see Fig. 7.11). Influences of the state of the boundary layer (laminar or turbulent), the gas model, radiation cooling, and the location (windward side/leeward side) on the wall temperature. H2K-conditions: wall temperature in the Navier-Stokes/RANS solution [43].

High-temperature real-gas effects cannot be neglected at M= 6.8 in the flight situation, at least regarding Tr. The switch from the perfect-gas model to the equilibrium real-gas model results in a drop of the recovery temperature by approximately 250 to 300 K for both laminar and turbulent flow.

If we switch on radiation cooling (є = 0.85), the picture changes drasti­cally. The wall temperature is now the radiation-adiabatic temperature. In the case of laminar flow it drops from the stagnation-point temperature, now Tw = Tra « 1,600 K, very fast to temperatures between 600 K and 500 K. This drop follows quite well the proportionality Tw ж x-0’125 on flat surface portions, see eq. (3.25) and also Table 7.7. It is due to the inverse of the growth of the laminar boundary-layer thickness (ж x0’5), Table 7.3, and to the fact, that the surface-radiation flux is proportional to T^.

The case of turbulent flow reveals at once that the radiation-adiabatic temperature reacts much stronger on the state of the boundary layer, laminar or turbulent, than the adiabatic temperature. In our case we have an increase

of Tw (= Tra) behind the location of laminar-turbulent transition by about 400 K.

The transition location was chosen arbitrarily to lie between approxi­mately x/L = 0.08 and 0.12.[115] In reality a transition zone of larger extent in the x-direction is present with different positions at the windward and the lee­ward side, and with possible “tongues” extending upstream or downstream. (See in this regard for instance [1].)

The drop of the radiation-adiabatic wall temperature in the case of tur­bulent flow is weaker than for laminar flow. It follows quite well the propor­tionality Tw ж x-0 05, eq. (3.25), see also Table 7.7. This is due to the inverse of the growth of the thickness of the viscous sub-layer, respectively of the turbulent scaling length, of the turbulent boundary-layer, ж x0 2, Table 7.3, and of course also to the fact, that the surface-radiation flux is proportional

to TW.

In Fig. 7.10 also the radiation-adiabatic temperatures at the upper sym­metry line (leeward side of the forebody) are indicated. Qualitatively they behave like those on the windward side, however, the data for laminar flow are approximately 80 K lower, and for turbulent flow even 200 K lower than on the windward side. We observe that here the increase behind the laminar – turbulent transition zone is not as severe as on the windward side.

The explanation for the different temperature levels at the windward and the leeward side lies with the different unit Reynolds numbers at the two sides, and hence with the different boundary-layer thicknesses. We show this with data found with the help of an approximation of the vehicle by an infinitely thin flat plate. The ratio of the unit Reynolds numbers at the leeward (Ref ) and at the windward (ReW) symmetry lines at x/L = 0.5 for the flight Mach number Mо =6.8 and the angle of attack a = 6°, as well as the computed and the approximatively scaled, eq. (3.34), temperatures are given in Table 7.10.

Table 7.10. Computed and approximatively scaled radiation-adiabatic tempera­ture ratios (leeward (‘l’) side to windward (‘w’) side) at x/L = 0.5 on the symmetry lines of the SANGER forebody at M= 6.8 and a = 6°. Ref/Rel Tra, l/Tra, w laminar Tra, l /Tra, w turbulent Computed data, Fig. 7.10 – ra 0.82 ra 0.76 Scaled data, eq. (3.34) ss 0.368 (flat plate) ra 0.88 ra 0.84

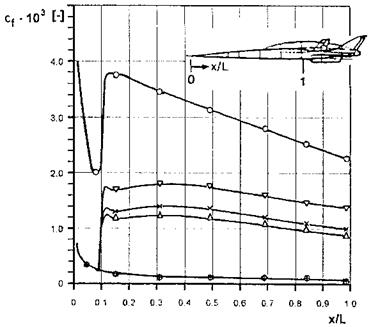
The skin-friction coefficient along the symmetry line of the windward side of the forebody is given in Fig. 7.11. The influence of the wall temperature on the skin friction is very small, if the boundary layer is laminar, Table 7.5. The skin-friction coefficient drop follows well the primary proportionality rw a x-0 5, eq. (7.146), see also Table 7.5. This is due to the inverse of the growth of the laminar boundary-layer thickness, a x0 5, Table 7.3.

Fig. 7.11. n coefficients along the lower symmetry line of the forebody of the lower stage of SANGER in the flight and the wind-tunnel (H2K) situation [41, 42] (symbols see Fig. 7.10). Influences of the state of the boundary layer (laminar or turbulent), the gas model, and radiation cooling on the skin-friction coefficient (windward side only). H2K-data: Navier-Stokes/RANS solution [43].

The influence of the wall temperature on the skin-friction coefficient in the cases of turbulent flow is very large. We note first for all three cases a non­monotonic behavior of cf behind the transition location in the downstream direction. The cause of this behavior is not clear. It can be the reaction on the enforced transition of the simple algebraic turbulence model employed in [42], as well as three-dimensional effects and also possible resolution problems of the discretization for the numerical solution. Only for x/L ^ 0.5 the skin – friction coefficient drops approximately proportional to x-0 2, which is the primary proportionality for turbulent flow, Table 7.5.

Important is the observation, that the turbulent skin friction is lowest for the perfect gas, adiabatic (є = 0) wall case, and largest (approximately 45 per cent larger) for the radiation-cooling (є = 0.85) case. For the equilibrium gas, adiabatic wall case cf is somewhat larger than for the perfect gas, adiabatic wall case, i. e., high-temperature real-gas effects can play a non-negligible role regarding turbulent skin friction.

The general result is: the smaller the wall temperature, the larger is the skin-friction of a turbulent boundary layer. This behavior follows with good approximation the dependence tw ж (T*/Tref )"(1+ш)-1, eq. (7.146), see also Table 7.5.

We give cf-ratios computed at x/L = 0.5 in Table 7.11. We compare them with data found with the simple relation for flat surface portions with reference-temperature extension, eq. (7.146). This is done with a scaling by means of T*/Tref. The ratio T*/Tref, eq. (7.70), is computed with Tw taken from Fig. 7.10, with Ye = 1.4, Me = M= 6.8, and rturb = 0.896. We find at x/L = 0.5 that the skin-friction coefficient at the radiation-adiabatic surface is approximately 1.45 times larger than at the adiabatic surface with perfect gas assumption, and approximately 1.3 times at the adiabatic surface with equilibrium real-gas assumption. The scaled data are in fair agreement with the computed data. The scaling of the skin-friction coefficient for laminar flow gives an influence of the wall temperature of less than 10 per cent, which is barely discernible in the data plotted in Fig. 7.11.

Table 7.11. Computed and scaled turbulent skin-friction ratios at the windward side at x/L = 0.5 of the SANGER forebody at M= 6.8 and a = 6°. ‘a’ denotes the case with perfect gas, e = 0 (Л), ‘b’ the case with real gas, e = 0 (x), and ‘c’ the case with real gas, e = 0.85 (v), Fig. 7.10. Cf, c/Cf, a Cf, c/Cffi Computed data, Fig. 7.11 ra 1.477 ra 1.286 Scaled data, eq. (7.146) ra 1.356 ra 1.296

The results in general show that for the estimation of the skin-friction drag of CAV’s the surface temperature, which is governed predominantly by radiation cooling, must be properly taken into account, at least for turbulent flow. In view of the fact that the skin-friction drag may account for a large part of the total drag, the vehicle surface should be flown as hot as possible. However, one has to take into account possible side effects: rise of the pressure (form) drag, degradation of the effectiveness of aerodynamic trim and control surfaces, Section 10.7.

For a typical cold hypersonic wind-tunnel situation, that of the H2K at DLR Koln-Porz, Germany, a computation has been performed for the

SANGER forebody, too [43]. The Mach number was the same as the flight Mach number, but the flow parameters were different, and in particular the surface temperature Tw was the typical ambient model temperature, Table 7.9. The resulting skin friction is quantitatively and qualitatively vastly dif­ferent from that computed for the flight situation. In the laminar regime just ahead of the location of enforced transition it is about eight times larger than that computed for the flight situation. The data do not scale properly.

In the turbulent regime the skin friction initially is about 2.2 times larger than for the flight situation. The scaling yields 2.65, which is a reasonable result. The slope of cf in the turbulent regime is much steeper in the wind- tunnel situation than in the flight situation. Both the unit Reynolds number, and the small body length may play a role in so far, as transition was enforced at a local Reynolds number which is too small to sustain turbulence. (In the wind tunnel experiment transition was not enforced and the state of the boundary layer was not clearly established.)

The large influence of the state of the boundary layer, laminar or tur­bulent, on the wall temperature and on the skin friction, if the surface is radiation cooled, poses big problems in hypersonic flight vehicle design. The transition location is very important in view of thermal-surface effects, as well as of thermal loads. The prediction and verification of the viscous drag (skin-friction drag plus viscosity-induced pressure drag (form drag)) is very problematic, if the flight vehicle is drag critical, which in general holds for CAV’s. Fig. 7.11 indicates that in such cases with present-day wind-tunnel techniques, especially with cold model surfaces, the viscous drag cannot be found with the needed degree of accuracy and reliability.

7.3 Problems

Problem 7.1. The flow past a flat plate has a unit Reynolds number ReU = 106 m-1. Assume incompressible flow and determine on the plate at x = 1 m for a) laminar and b) turbulent flow the boundary-layer thicknesses 5, 5i, 52, the shape factors H12, and for turbulent flow in addition 5vs, and 5sc.

Problem 7.2. The flow past a flat plate is that of Problem 7.1. Assume incompressible flow and determine on the plate, but now at x = 10 m for a) laminar and b) turbulent flow the boundary-layer thicknesses 5, 51, 52, the shape factors H12, and for turbulent flow in addition 5vs, and 5sc.

Problem 7.3. Compare and discuss the results from Problem 7.1 and 7.2 in view of Table 7.3.

Problem 7.4. Derive the formula (perfect gas) for the skin-friction drag Df (with reference-temperature extension) of one side of a flat plate with the length L and the width b (the reference area is Aref = bL). Write the formula for both fully laminar and fully turbulent flow.

Problem 7.5. Compute the skin-friction drag Df of a flat plate at zero angle of attack (both sides wetted!). The parameters are similar to those in Problem 6.8: Mсо = 6, H = 30 km, Aref = 1,860 m2, Lref = 80 m. Disregard possible hypersonic viscous interaction, Section 9.3. Use the power-law relation, eq. (4.15), for the viscosity, take in the relation for the reference temperature, eq. (7.70), у = 1.4, Pr* = 0.74. Assume laminar flow and two wall temperatures:

a) Tw = 1,000 K, b) Tw = 2,000 K.

Problem 7.6. Compute the skin-friction drag Df of a flat plate at zero angle of attack like in Problem 7.5, but now for turbulent flow. Mind the different recovery factors of laminar and turbulent flow.

Problem 7.7. Discuss the results from Problem 7.5 and 7.6, in particular also the influence of the wall temperature on the skin-friction drag. How large is the ratio ‘turbulent drag’ to ‘laminar drag’ for a) the lower temperature,

b) the higher temperature? How much in per cent is the drag reduced for the higher temperature for a) laminar flow, b) turbulent flow?

Problem 7.8. Compute the skin-friction forces Lf and Df for the CAV of Problem 6.8. On the windward (w) side we have the boundary-layer edge data: Mw, e = 5.182, ReW e = 3.123-106 m-1, vw, e = 1,773.63 m/s, pw, e = 0.0327 kg/m3, Tw, e = 291.52 K, and on the leeward (l) side: Mle = 6.997, Refe = 1.294-106’m-1, v, e = 1,840.01 m/s, p, e = 0.00297 kg/m3, Tle = 172.12 K. Consider each side separately as flat plate surface. Assume fully turbulent flow, wall temperatures Tw, w = 1,000 K, Tl, w = 800 K, and the other parameters like in Problem 7.6.

Problem 7.9. Add the inviscid parts, Problem 6.8, and the skin-friction parts, Problem 7.8, of the lift and the drag of the CAV, compute the lift to drag ratio and discuss the results.