DIRECTIONAL CHARACTERISTICS OF AIRPLANES

In considering the directional characteristics of airplanes it is assumed that the wings are kept horizontal by a suitable deflection of the ailerons. Thus, the vertical axis may be isolated from those in the other two directions. The static stability of such motions is then of the same type as that of a weather vane (“weather cock”). Subjects discussed in this chapter are: yaw moments due to fuselage and engine nacelles, stabilizing and control characteristics of the vertical tail and the influence of the propulsion system on yaw moments. Yaw moments arising in the wing (in connection with roll moments) are presented in Chapter XIV on lateral stability.

I. GENERAL

“Yawing” is meant to indicate rotation about the normal (or vertical axis). The angle of yaw indicates the

angular displacement about that axis, of the airplane in relation to the wind. In contradistinction, sideslipping means a lateral displacement of an airplane, without rotation, as in figure 1. However, to get into a sideslip, the pilot has to yaw his airplane first. As far as the usual wind tunnel tests are concerned, the models are not “yawing” at all. The term “yawing” moment thus seems to be misleading. Actually, static forces and moments are measured at an angle of yaw, or in sideslipping condition. It thus seems to be better to call this type of moment “yaw moment”. In a dynamic analysis of motions about the normal axis, there is also a damping moment due to yawing. This moment will be discussed in the dynamic part of Chapter XIV dealing with “lateral stability”.

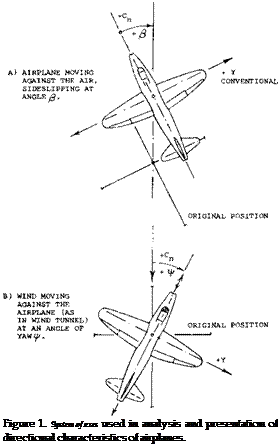
Reference. The system of axes used in this chapter is illustrated in figure 1. The angle of yaw ” and/or of sideslipping due to yaw or when sideslipping, are

defined about the vertical or lift axis, while the “lateral” force is normal to the fuselage axis. There is a convention whereby forces and moments are designated to be positive when their direction coincides with “positive” displacement of the airplane, that is a motion to the right is positive as seen by the pilot. It must be noted, however, that the angles /3 and ^ have opposite signs. A stabilizing yaw moment (“N”) produced by a vertical tail-surface would thus be represented by a positive value of the derivative d“N”/d/^, or a negative value of d“N”/d^ , while the derivative of the lateral force in the tail responsible for the moment would have the opposite sign in either case. To avoid any misunderstanding, it is recommended to think in terms of “stabilizing” or “destabilizing”, regardless of the direction (right or left) into which the airplane may turn.

Yaw Moments arising in conventional airplane configurations, are as follows:

a) due to fuselage and nacelles, usually destabilizing;

b) stabilizing moment provided by the vertical tail surface;

c) a destabilizing contribution due to propeller normal force, (see figure 3 chapter XII)

The yaw moment derivative of the wing can theoretically be zero. In reality, a stabilizing component is associated with the roll moment which every airplane requires for lateral stability, chapter XIV. In the discussion of direc­tional stability in this chapter, it is assumed that any roll moment be eliminated (balanced) by aileron deflection. In short, the wing is assumed not to contribute to the yaw moment of the airplane.

Notation. The notation specifically applying to directional characteristics is as follows:

Y = lateral force; “N” = yaw moment

where “N” (indicating the “normal” axis about which the moment is defined) should not be confused with N, de­noting “normal force”. Non-dimensional coefficients are

С у = Y/qS; and Cn = “N”/qSb (1)

where S = wing area and b = wing span of the airplane considered. Usually, and at least within the range of lift coefficients below stalling, the lateral force as well as the yaw moment vary in proportion to the angle of sideslip­ping. The most convenient way to describe this variation is by means of the derivatives

Су/з = dCy jdp; and С-ті/З = dC^/d/5 (2)

where /5 = angle of sideslipping, in degrees. In airplanes, the axis about which the yaw moment is taken, is usually the vertical (or the normal) through the CG.

Static Stability. Like the longitudinal case an airplane will have or will be statically stable when it has the tendency to return to its direction of flight after being disturbed. Although there is always an interacting roll effect it is possible to assume proper compensation so that the yaw stability is treated separately. Thus if the airplane has static stability in yaw a moment must be generated from the displacement that will tend to return it to a zero slideslip. Thus in the stability axis of notation dQv/dfi must be positive, figure 1, if the airplane is to be statically stable in yaw.