Features of a Linearized Formulation and an Algorithm of the Solution

d[9]p d2p d2p dx2 dy2 dz2

A linear formulation for the perturbed velocity potential of absolute motion can be easily derived from the nonlinear formulation in the form

Signs “plus” and “minus” correspond to the upper and lower surfaces. • Flow tangency condition on the ground:

• Dynamic condition of pressure continuity across the wake: P~ =P+, У = h, (x, z) Є W

or

so that p = p{x, у, z, t) and rewrite (3.6) as

Equation (3.8) can be demonstrated to be identical to the following set of equations:

ip+(x, h,z, t) – ip (x, h,z, t)=(p+(xte, h,z,0)~<p (xte, h,z, 0),

(x, z)eW, x-xte{z)+t = 0, (3.9)

where xte = Xte(^) is the equation of the trailing edge in the adopted coordinate system.[10] In terms of “physical” time £,

f U(t)dt = 0. Jt[11]

ip+(x, h,z, t) – <p (x, h,z, t) = ip+(xte, h,z, t*) -<p (xte, h,z, t*),

In fact, the alternative forms (3.9),(3.10) of the dynamic boundary condi­tion (3.5) in the wake correspond to the Kelvin (Thomson) theorem and express the jump of the velocity potential across the vortex sheet at an ar­bitrary point of the latter, if this jump is known at any time at the trailing edge of the wing. The Kutta-Zhukovsky condition at the trailing edge can be viewed as incorporated into the above dynamic condition in the wake.

• The continuity of the vertical component of the flow velocity across the wake:

Linearizing the kinematic condition (2.5), we derive

• Decay of perturbations at infinity:

x2 + y2 + z2 oo. (3.12)

In channel flow, the same asymptotic expansion (2.11), as earlier, can be utilized, although in the linear case

= оф«1. (3.13)

Using the linearized version of the procedures, demonstrated in 2.2, one can show that the channel flow equation is identical to the Poisson equation

where h* = h*/h = y — yg = 1 + О (є/ft), whereas

(3.15)

For channel flow under the wake a similar equation holds, but in this case it has to be solved with respect to the induced downwash

The components of the induced downwash in the wake can be determined by a linearized version of (2.24), namely,

U(t*)dt* U(t*)dt

Using the dynamic condition in the wake in the Kelvin (Thomson) form (3.10), one can calculate aw as

where t* is related to x and t by equation (3.10).

For a steady flow with asymptotic error О (ft), the downwash in the wake is not dependent on x. With this in mind, determination of aw ~ aWl becomes still simpler:

_ _ <92

cyw 00 cyWl = ^^2 <Ph z)’ (3.19)

The flow above the lifting system and its wake (upper flow) is identical to what was considered previously. The upper flow potential (fu is of the order of 0(e) and, to the lowest order, is described by an expansion

in which (pUl is represented by (2.31) and has the “edge” asymptotics given by (2.32) and (2.33).

Local flows are linearized consistent with the overall linearization scheme. Stretching of local coordinates is performed by the ground clearance h rather than the local instantaneous distance /і* of the edge from the ground as in the nonlinear case. Otherwise, all previous results of section 2 hold for the linear case.

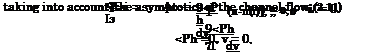The boundary condition for the channel flow equation (3.14) at the leading (side) edge li for an infinitely thin edge can be derived from (2.73) setting hi = 1. Then

The boundary condition for the channel flow equation (3.14) at the trailing edge /2 for a sharp straight edge can be derived from (2.98) setting h* = 1. Then

Pi* = 2

The coefficients of the lift and moments are given by formulas (2.104)—(2.106), but the pressures on the upper and lower surfaces of the wing are both cal­culated by using linear differential operators

Within the linearized formulation, the extreme ground effect case has a still simpler mathematical description than in the nonlinear theory. Correspond­ing relationships have the form

Transition points, separating the leading (side) and trailing edges, are coin­cident with the wing tips.