Our heavyweight helicopter equal in the world does not have
In Rostov started production of the most load-lifting rotary-wing car The Russian holding «Helicopt[...]
Arbitrary Motion of a Profile in Subsonic Flow
For the case of compressible flow response of an airfoil to the arbitrary motion differs from that of the incompressible flow. Therefore, we have to modify the
|
M |
b0 |
b1 |
b2 |
Ьз |
b1 |
b2 |
Ьз |
|
|
/(s) |
0 |
1.0 |
0.165 |
0.335 |
0 |
0.0455 |
0.3 |
– |
|
0.5 |
1.155 |
0.406 |
0.249 |
-0.773 |
0.0753 |
0.372 |
1.89 |
|
|
0.6 |
1.25 |
0.452 |
0.63 |
-0.893 |
0.0646 |
0.481 |
0.958 |
|
|
0.7 |
1.4 |
0.5096 |
0.567 |
-0.5866 |
0.0536 |
0.357 |
0.902 |
|
|
Z(s) |
0 |
1.0 |
0.5 |
0.5 |
0 |
0.13 |
1.0 |
– |
|
0.5 |
1.155 |
0.45 |
0.47 |
0.235 |
0.0716 |
0.374 |
2.165 |
|
|
0.6 |
1.25 |
0.41 |
0.538 |
0.302 |
0.0545 |
0.257 |
1.461 |
|
|
0.7 |
1.4 |
0.563 |
0.645 |
0.192 |
0.0542 |
0.3125 |
1.474 |
|
Table 5.1 Variations of Wagner an Kussner functions with respect to the Mach number of the flow |
indicial admittance functions in terms of the Mach number of the flow for the sudden angle of attack change and for the gust impingement problems. Similar to that of incompressible flow we denote the Wagner function, u(s), for the sudden angle of attack change, ao, and the Kussner function, v(s), for the gust effects to give with the Wagner function
cl {s)=2na, o u(s). (5.54)
For the gust of intensity wo we write with the Kussner function
w
CLg (s) = 2%a. o-U v(s) (5.55)
Here, s denotes the reduced time based on the half chord of the airfoil. For the Wagner and the Kussner functions we have the following general approximation in terms of the exponential functions as follows (Bisplinghoff et al. 1996).
^( ) = bo — b1 e—b1s — b2e—b2 — b3e—Ьзs
v(s)
 |
 |
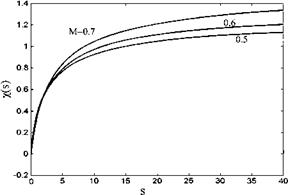The values for the exponents of each function with respect to the Mach number are given in Table 5.1. Figure 5.8 gives the plots for the Kussner function in terms
of three different Mach numbers. The compressibility effect in these plots is evident as the steady state is reached.
