Other problems of turning

In order to get into a turn the pilot puts on bank by means of the ailerons, but once the turn has commenced the outer wing will be travelling faster than the inner wing and will therefore obtain more lift, so he may find that not only is it necessary to take off the aileron control but actually to apply opposite aileron by moving the control column against the direction of bank – this is called holding off bank.

An interesting point is that this effect is different in turns on a glide and on a climb. On a gliding turn the whole aircraft will move the same distance downwards during one complete turn, but the inner wing, because it is turning on a smaller radius, will have descended on a steeper spiral than the outer wing; therefore the air will have come up to meet it at a steeper angle, in other words the inner wing will have a larger angle of attack and so obtain more lift than the outer wing. The extra lift obtained in this way may compensate, or more than compensate, the lift obtained by the outer wing due to increase in velocity. Thus in a gliding turn there may be little or no need to hold off bank.

In a climbing turn, on the other hand, the inner wing still describes a steeper spiral, but this time it is an upward spiral, so the air comes down to meet the inner wing more than the outer wing, thus reducing the angle of attack on the inner wing. So, in this case, the outer wing has more lift both because of vel­ocity and because of increased angle, and there is even more necessity for holding off bank than during a normal turn.

Another interesting way of looking at the problem of gliding and climbing turns is to analyse the motion of an aircraft around its three axes during such turns. In a flat turn, i. e. a level turn without any bank, the aircraft is yawing only. In a banked level turn, the aircraft is yawing and pitching – in the extreme of a vertically banked turn it would be pitching only. But in a gliding or climbing turn the aircraft is pitching, yawing and rolling. In a gliding turn it is rolling
inwards; in a climbing turn, outwards. The inward roll of the gliding turn causes the extra angle of attack on the inner wing, the outward roll of the climbing turn on the outer wing. Many people find it difficult to believe this. If the reader is in such difficulty conviction may come from one of two methods; which will suit best will depend upon the reader’s temperament. The mathematically – minded may like to analyse the motion in terms of the following (Fig. 8.7) –

The rate of turn of the complete aeroplane (about the vertical), Q.

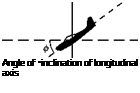
The angle of bank of the aeroplane, в.

The angle of pitch of the aeroplane, ф.

A little thought will reveal the fact that the

Rate of yaw = Q. cos ф. cos в.

Rate of pitch = Q. cos ф. sin в.

Rate of roll = £2 . sin ф.

Translating this back into English, and taking one of the extreme examples, when в = 0, i. e. no bank, and 0 = 0, i. e. no pitch, cos в and cos ф will be 1, sin в and sin ф will be 0.

.’. rate of yaw = £2 = rate of turn of complete aeroplane.

Rate of pitch and rate of roll are zero. All of which we had previously decided for the flat turn.

The reader (mathematically-minded) may like to work out the other extremes such as the vertical bank (в = 90°) or vertical pitch (ф = 90°), or better still the more real cases with reasonable values of в and ф.

Fig 8.7 Gliding turns

Notice that the rate of roll depends entirely on the angle of pitch, i. e. the inclination of the longitudinal axis to the vertical – if this is zero, there is no rate of roll even though the aircraft may be descending or climbing.

What about the reader who does not like mathematics? Get hold of a model aeroplane, or, failing this, a waste-paper basket and spend a few minutes making it do upward and downward spirals; some people are convinced by doing gliding and climbing turns with their hand and wrist – and their friends may be amused in watching!

At large angles of bank there is less difference in velocity, and in angle, between inner and outer wings, and so the question of holding off bank becomes less important; but much more difficult problems arise to take its place.

First, though, let us go back to the other extreme and consider what is called a ‘flat turn’, i. e. one that is all yaw and without any bank at all.

Very slight turns of this kind have sometimes been useful when approaching a target for bombing purposes, but otherwise they are in the nature of ‘crazy flying’, in other words, incorrect flying, and good pilots always try to keep their sideslip indicator in the central position. Actually flat turns are rather dif­ficult to execute for several reasons. First, the extra velocity of the other wing tends to bank the aeroplane automatically; secondly, the lateral stability (explained later) acts in such a way as to try to prevent the outward skid by banking the aeroplane; thirdly, the side area is often insufficient to provide enough inward force to cause a turn except on a very large radius; fourthly, the directional stability (also explained later) opposes the action of the rudder and tends to put the nose of the aircraft back so that it will continue on a straight path. Taking these four reasons together, it will be realised that an aeroplane has a strong objection to a flat turn!

Modern aircraft have a small side surface and if this is coupled with good directional stability, for the last two reasons particularly, a flat turn becomes virtually impossible. So much is this so that it is very little use applying rudder to start a turn, the correct technique being to put on bank only.