Total pressure change across the normal shock

From the above sections it can be seen that a finite entropy increase occurs in the flow across a shock wave, implying that a degradation of energy takes place. Since, in the flow as a whole, no heat is acquired or lost the total temperature (total enthalpy) is constant and the dissipation manifests itself as a loss in total pressure. Total pressure is defined as the pressure obtained by bringing gas to rest isentropically.

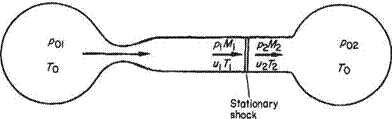
Now the model flow of a uniform stream of gas of unit area flowing through a shock is extended upstream, by assuming the gas to have acquired the conditions of suffix 1 by expansion from a reservoir of pressure poi and temperature To, and downstream, by bringing the gas to rest isentropically to a total pressurepo2 (Fig. 6.8)

Isentropic flow from the upstream reservoir to just ahead of the shock gives, from Eqn (6.18a):

Fig. 6.8

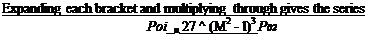(7+1)2 3.

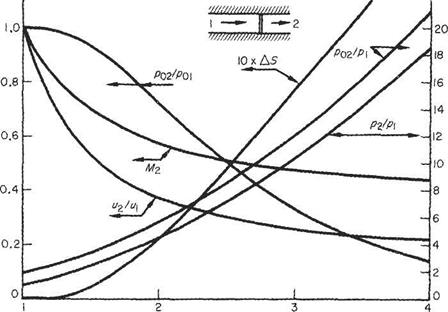
For values of Mach number close to unity (but greater than unity) the sum of the terms involving M2 is small and very close to the value of the first term shown, so that the proportional change in total pressure through the shock wave is

Apo рої – poi _ 27 (Mj – l)3

Poi Po (7+1)2 3

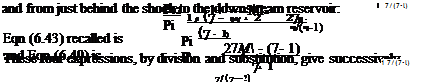
It can be deduced from the curve (Fig. 6.9) that this quantity increases only slowly from zero near Mi = 1, so that the same argument for ignoring the entropy increase (Section 6.4.6) applies here. Since from entropy considerations M> , Eqn (6.55) shows that the total pressure always drops through a shock wave. The two phenomena,

Mi Fig. 6.9

i. e. total pressure drop and entropy increase, are in fact related, as may be seen in the following.

Recalling Eqn (1.32) for entropy:

eAs/c, ^Pi (Рі_Рча (Pm Pi KpiJ Poi P02

since

etc.

Pi Яві ‘

But across the shock To is constant and, therefore, from the equation of state Poi/Poi — Р02ІР02 and entropy becomes

7-1

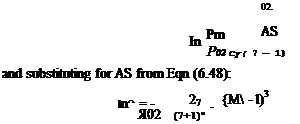Now for values of Mi near unity /З 1 and

A/>o __ />Q1 -/>02 _ ^ _ />01 />01