Unsteady Aerodynamics for Trailing-Edge Scattering

Most attached flows over well designed airfoils with a more or less sharp trailing edge belong the regime illustrated in Fig. 9. Turbulence carried in the boundary layers radiates sound because it is rapidly re-organized around the trailing-edge. Analytical sound predictions require a special mathematical statement. They are better derived starting from the incident wall-pressure field corresponding to the boundary-layer turbulence, since the Kutta condition is expressed in terms of a pressure release. In that sense the convection speed Uc of Fig. 9 refers to the wall-pressure trace of the incident vortex dynamics rather than to the incriminated velocity field. The sound-generating mechanism is addressed by the generic configuration of hydrodynamic pressure patterns convected past the edge of a thin rigid plate, sketched in Fig. 10.

More precisely the wall-pressure field is again Fourier-analyzed and ex­pressed as a combination of pressure gusts, and the airfoil response deduced for each gust. This suggests that few differences arise in the modeling with

Figure 9. Pure trailing-edge noise configuration. The sec­ondary convection speed U C in the wake introduced by Howe (1978) is not used in the present modeling approach.

respect to the previous mechanism, except that what was done with the velocity or the velocity potential is now repeated with the disturbance pres­sure. It must be noted however that different models have been proposed in the literature (see for instance Howe (1978) and Blake (1986)). They are not detailed here.

The incident forcing by a wall-pressure gust of amplitude, say P0 is con­sidered for one side only, that most often is the suction side of a loaded airfoil. The proper way of imposing a Kutta condition remains a matter of controversy when resorting to simplified models expected to reproduce real-life flows. A full Kutta condition on the pressure jump is most often considered. The scattered pressure field around the edge involves contri­butions in phase opposition on each side of the plate, so that the pressure jump is continuous and zero. Another choice is to assume that an incident vortical pattern in the boundary layer tends to follow its path in the wake. According to this interpretation discussed by Moreau & Roger (2009), the disturbance pressure remains continuous and zero around the edge instead of the pressure jump, and a counter-pressure of amplitude P0 is distributed on each side of the airfoil. A factor 2 makes the difference between both assumptions, and the effect will be 6 dB more in the far-field noise with the second one, for the same incident turbulence. Apart from that, the proce­dure will be declined in the same way. According to authors’ experience with a set of airfoils tested at low speeds, the condition of zero pressure around the edge produces the best agreement with measured data; further-

x

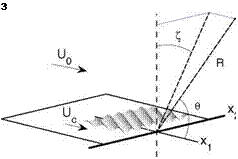
Figure 10. Reference frame for trailing-edge noise model­ing, and schematic view of a wall-pressure gust. Radiation angles mentioned for completeness. в is used in asymptotic formulations of section 6.1.

more it ensures that Amiet’s formulation coincides with asymptotic Howe’s theory at very high non-dimensional frequencies (Moreau & Roger (2009)).

In the derivations the origin of coordinates is taken at trailing-edge mid­point according to the sketch of Fig. 10. Pressure gusts are defined in a first step as if there were neither scattering nor edge. Though turbulence in a boundary layer is not homogeneous in the stream-wise direction, it is assumed almost homogeneous over the small extent just upstream of the edge where the dominant vortex dynamics takes place. More rigorously a gust should be given a growing amplitude, which would be equivalent to adding an imaginary part to the stream-wise wavenumber. Accepting the simplification, a gust of wall-pressure P0 is then forced to zero at the trailing edge by adding a counter-pressure Pi in phase opposition on both sides of the airfoil (this ensures continuity of the pressure in a close vicinity of the edge). If the airfoil leading edge is removed to infinity, Pi is solution of a Schwarzschild’s problem since it has to exactly cancel P0 in the wake and have a zero normal derivative upstream. The corresponding induced lift does not satisfy the condition of zero potential upstream of the airfoil leading edge. In the original formulation proposed by Amiet (1976-b), this is not considered a drawback because trailing-edge noise generally involves
small-scale turbulence and relatively high frequencies for which the induced counter-pressure decreases rapidly away from the edge. For low-frequency needs, a leading-edge back-scattering correction can be derived by going back to the disturbance potential and performing another application of Schwarzschild’s theorem (Roger & Moreau (2005)). The back-scattering correction is not negligible but is is only significant in limited cases. As such it is not detailed here. In contrast the duality of supercritical and sub-critical gusts still makes sense in the three-dimensional formulation of trailing-edge noise. The induced lift of supercritical gusts is £ = £1 + £2, with the dominant contribution £ ~ £ given as

2 ei (akt y1+ k* УІ) [(! _ ■) E (- [ak* + к + Mo j y2) _ 1 ,

(9)

where a = U0/Uc is the ratio of the free-stream velocity to the averaged convection speed of the incident boundary-layer wall-pressure disturbances. For sub-critical gusts, the expression reads

with the same other notations as in previous section.

It is worth noting that the model assumptions remain questionable, prob­ably because the physical processes highly depend on the flow features and airfoil design in the vicinity of the trailing edge. Reducing the airfoil to a flat plate of zero thickness and assuming locally homogeneous turbulence is a concern if the actual shape is not much thinner than the boundary-layers, as in the case of quite thick beveled edges. In particular, the meaning and the hypothesis of a full or partial Kutta condition, as well as questions about the coupled incident and scattered wall pressures have been examined by some authors (Howe (1978), Zhou & Joseph (2007)). This variability of small-scale motions in boundary-layers explains both the difficulty of defin­ing universal scaling laws, addressed in section 6.1, and the either successful or disappointing comparisons of model predictions with measurements. It must be also kept in mind that trailing-edge noise modeling is addressed for a single incriminated boundary layer. Most often this one is the suction – side boundary layer of a loaded airfoil; if the pressure-side boundary layer is also turbulent, the model must be considered twice with different param­eters, assuming uncoupled sides. Outdoor measurements of the trailing – edge noise of wind-turbine blades reported by Oerlemans & Mendez-Lopez (2005) exhibit spectral features involving both sides of the blades: logically the high-frequency range is attributed to the thinner boundary layers of the
pressure sides whereas the low-and-middle frequency range is attributed to the thicker suction-side boundary layers.

Chord-wise radiation integrals (aeroacoustic transfer functions) are also derived from the unsteady lift distributions, following the same methodology as in section 2.5. The expression initially proposed by Amiet (1976-b) for parallel gusts has been readdressed by Roger & Moreau (2005) to account for three-dimensionality. For supercritical gusts the result reads

e-2iC ( j B

I = j(1 – i) e2tCI ^zrc E [2(B – C)] – (1 – i) E [2B] + 1

(11)

with B = ak + M0 p + к, C = ak + p (M0 — x/S0). Again for numerical issues it is better implemented using the function ES. For sub-critical gusts the radiation integral is found as

II = |(1 – i) e2iC VW’ES [2 (B’ – C)] – Ф(0) (j-2iB’]1/2^ +1|

І (12)

with B’ = ak + ік’ + M0 p.

Because trailing-edge scattering and turbulence impingement involve vortex dynamics close to an edge, the associated radiation properties have some similarities. Typically, isolated oblique supercritical wall-pressure gusts force trailing-edge noise in oblique directions, in the same way as illustrated in Fig. 8 for turbulence-impingement noise but with lobes now pointing preferentially upstream.