Aerodynamic Strip Theory

Thus far, we examine rapid-solution methods using panels and we see that we can quickly obtain a good estimate of the overall inviscid performance (i. e., lift coeffi­cient, induced-drag coefficient, and moment coefficient) of any candidate wing. We

can even obtain rather good spanwise information regarding the variation of sev­eral quantities, such as induced angle of attack and downwash. However, chordwise information is limited. In the wing-panel methods, we obtain information only at a few chordwise stations corresponding to locations of the singularities; in lifting-line theory, there is no chordwise information at all. As discussed in Chapter 8, such information is necessary to determine the effects of the boundary layer to compute the viscous drag. Chapter 8 also points out that the local surface-pressure gradient has a key role in the behavior of the boundary layer and that it should be known accurately. Thus, we discuss here a relatively fast method of supplementing the information from a panel method, or even from lifting-line theory, to obtain such detailed information. Using the effective angle-of-attack information from either a three-dimensional panel code or lifting-line theory, aerodynamic strip theory describes the chordwise pressure distribution on a three-dimensional wing by com­puting the chordwise pressure distribution at separate spanwise stations. Because this approach necessarily neglects spanwise flow, the method works best on high AR wings or wings with little sweep. Notice that the three-dimensional effect is not com­pletely eliminated because we use an effective angle of attack that was determined by accounting for three-dimensional effects.

Virtually any three-dimensional panel method combined with any airfoil pressure-distribution method can be used in this strip-theory approach. Here, we demonstrate the idea by using the VLM and the AIRFOIL codes introduced previ­ously. The basic steps are as follows:

1. Use a three-dimensional panel code (e. g.,VLM) to compute the spanwise distri­bution of effective angle of attack, aeff, for a given wing. Recall from Eq. 6.13 that aeff = a – a;.

2. At selected spanwise stations, use the local wing-section information and the effective angle of attack to determine the section-pressure distribution

(i. e., airfoil).

Consider a straight-tapered wing with an AR of 6.4 and a taper ratio of 0.4; use the NACA 0012 airfoil as the wing section. Let the wing have a geometric angle of attack of 5° at the plane of symmetry and a linear twist (i. e., root to tip) of 3°. Using the VLM program illustrated previously, with 11 spanwise stations and 7 chordwise stations, the following results are obtained:

Spanwise Station, 2y/b aeff

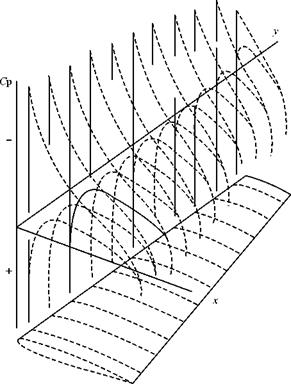For each spanwise station, the effective angle of attack is used in Program AIRFOIL, along with the section information, to obtain a detailed surface-pressure distribution at the station. The result is shown in Figure 6.25. Shown in the figure are the detailed pressure distributions from the Program AIRFOIL for each of the 11 spanwise stations used by the VLM program. The figure was generated by reading the 11 Cp data files generated by the Program AIRFOIL and making a plot of the wing and the Cp distributions. The Cp data files also would be used by a boundary – layer program to compute the viscous drag over the wing (see Chapter 8).