Application to swept wings

In the same way as for the incompressible case (see Section 5.7), the compressible flow over an infinite-span swept (or sheared) wing can be considered to be the superposition of two flows. One component is the flow perpendicular to the swept leading edge. The other is the flow parallel to the leading edge. The free-stream velocity now consists of two components, see Fig. 6.38. For the component perpen­dicular to the leading edge Eqn (6.118) becomes

Divide through by а2ж for the condition when V = a = a*, M^ etc.

and on substituting for p*jpoo in Eqn (6.134)

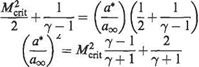(6.137)

Example 6.8 For the NACA 4 digit series of symmetrical aerofoil sections in incompressible flow the maximum disturbance velocity (u’/Foc)maJt (corresponding to (СД^п) varies in the following way with thickness-to-chord ratio, t/c:

NACA AEROFOIL DESIGNATION tic (косіша* NACA0006 0.06 0.107 NACA0008 0.08 0.133 NACA0010 0.10 0.158 NACA0012 0.12 0.188 NACA0015 0.15 0.233 NACA0018 0.18 0.278 NACA0021 0.21 0.325 NACA0024 0.24 0.374

Use this data to determine the critical Mach number for

(i) A straight wing of infinite span with a NACA 0010 wing section; and

(ii) An infinite-span wing with a 45° sweepback with the same wing section perpendicular to the leading edge.

All the 4-digit NACA wing sections are essentially the same shape, but with different thickness – to-chord ratios, as denoted by the last two digits. Thus a NACA 0010 wing section at a given freestream Mach number Mx is equivalent to a 4-digit NACA series in incompressible flow having a thickness of

(t/c)i = 0.100-MJ,

The maximum disturbance velocity, [(u//Koc)max]i for such a wing section is obtained by using linear interpolation on the data in the table given above. The maximum perturbation velocity in the actual compressible flow at Мх is given by

{d/VcxOma* — j _ [(“V^xOn

The maximum local Mach number is given approximately by

Equations (a) and (b) and linear interpolation of the table of data can be used to determine Мщах for a specified Moo• The results are set out in the table below

Linear interpolation between Moo = 0.75 and 0.8 gives the critical value of Mx ~ 0.78 (i. e. corresponding to Mmax = 1.0).

For the 45° swept-back wing

{t/c = 0.10^/1 – M£, cos2 Л = 0.100.0-0.5 M£,

Koo must be replaced by (Foo)„, i. e. Koo cos Л, so the maximum disturbance velocity is given by

The maximum local Mach number is then obtained from

Thus in a similar way as for the straight wing the following table is obtained.