Our heavyweight helicopter equal in the world does not have
In Rostov started production of the most load-lifting rotary-wing car The Russian holding «Helicopt[...]
Effect of Nose Bluntness—Entropy-Layer Instability
Configurations of hypersonic flight vehicles have blunt noses in order to cope with the large thermal loads there. We have seen in Sections 1.2 and 7.2 that the nose radius is important regarding the efficiency of radiation cooling. Nose bluntness on the other hand is the cause of the entropy layer, Sub-Section
6.4.2. This entropy layer is a shear layer, which is or is not swallowed by the boundary layer, depending on the nose radius and the Reynolds number of the flow case.[135]
It is justified to surmise that the entropy layer can play a role in laminar – turbulent transition of the boundary layer over blunt bodies. Experimental studies on slender cones have shown that this is the case, see, e. g., the overview and discussion in [11]. A small nose-tip bluntness increases the transition Reynolds number relative to that for a sharp-nosed cone. However, if the bluntness is increased further, this trend is reversed, and the transition Reynolds number decreases drastically.
Cone blunting locally decreases the boundary-layer edge Reynolds number, which partially explains the downstream movement of the transition location. The transition reversal is not yet fully understood, but entropy-layer instabilities appear to be a possible cause for it [39, 40].
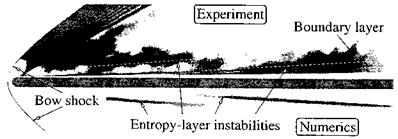
Recent experimental and theoretical studies by G. Dietz and S. Hein further support this view [41]. Their visualization of an entropy-layer instability on a flat plate with blunt leading edge is shown in Fig. 8.8. The oblique dark areas in the upper part indicate regions with large density gradients. These are most likely caused by instability waves in the entropy layer. If the entropy layer is swallowed, these disturbances finally are transported by convection into the boundary layer, where they interact with the boundary-layer disturbances.
|
Fig. 8.8. Entropy-layer instability in the flow past a flat plate with blunt leading edge: density gradients in direction normal to the wall in a Schlieren picture (upper part), compared to those of the perturbed flow, which were numerically obtained (lower part) [41]. Ыж = 2.5, ReP = 9.9-106 m-1, adiabatic surface, angle of attack a = 0°. The broken white lines in the Schlieren picture are the computed locations with maximum dp/dy, p being the density eigenfunction. |
Correlations of a large experimental data base regarding bluntness effects on flat plate transition are given in [42], where also effects of hypersonic viscous interaction, Section 9.3, are taken into account. A recent overview regarding work on entropy-layer instability can be found in [43].
