Our heavyweight helicopter equal in the world does not have
In Rostov started production of the most load-lifting rotary-wing car The Russian holding «Helicopt[...]
Near-field force calculation
 |
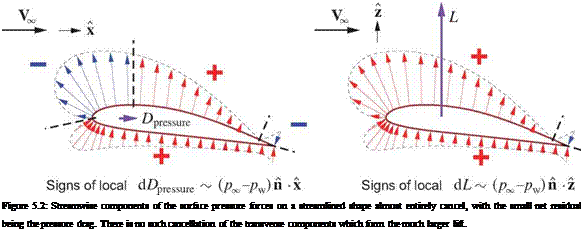In grid-based CFD solutions of the Navier-Stokes equations, the D, Y, L force components are calculated directly from the above definitions via numerical integration over the surface. But in simplified flow models, such as inviscid panel methods with or without viscous displacement models, this approach works only for the transverse Y, L components. If the streamwise D component were computed in this manner, it would be simply incorrect or very inaccurate. The main reason is that the Dpressure integral has relatively large positive and negative integrand contributions over the surface which mostly cancel, as shown in Figure 5.2.
In fact, for 2D inviscid flow the cancellation is theoretically perfect, which constitutes d’Alembert’s paradox.
D = Dpressure = 0 (2D inviscid flow) (5.9)
For 3D inviscid lifting flows the cancellation is not total, but is still extensive so that the pressure drag force is small. Even small errors in the surface pressures, or in the discrete integration method, then become very large when compared against the small (but crucial) remaining pressure drag.
The pressure-drag calculation difficulties remain for viscous flow simulations which employ the displacement effect. Such methods were discussed in Chapters 3 and 4, and two computed examples are shown in Figures 3.6 and 3.7. The modification of pw by the viscous displacement has the following effects:
• The modified lift L is now much more accurate than what’s predicted by the simple inviscid model. In particular, it can capture the effects of flow separation and stall as was shown in Figure 3.5.
• The modified Dpressure is now correctly nonzero in 2D flow. However, its accuracy is still relatively poor due to the streamwise-component pressure force cancellation shown in Figure 5.2. One reason is that errors associated with numerical integration over the discretized surface are still present. This is most severe for simple panel methods with relatively coarse paneling. Another reason is that even with the displacement effect corrections, the computed wall pressures pw still have residual errors. These may be small relative to lift, but are very significant relative to the much smaller pressure drag. These errors in pw are caused by the neglect of streamwise flow curvature in the viscous displacement-effect models, and are not easily removed.
