The equations of motion of a compressible fluid

The equation of continuity may be recalled in Cartesian coordinates for two-dimen­sional flow in the form

dp d(pu) d(pv) _ dt dx dy

since, in what follows, analysis of two-dimensional conditions is sufficient to demon­strate the method and derive valuable equations. The equations of motion may also be recalled in similar notation as

du du du 1 dp’

dt^ Udx^~ Vdy p dx

dv dv dv 1 dp

ch Udx^ Vdy p dy.

and for steady flow

Combining Eqns (6.114b) and (6.115a) gives an expression in terms of the local velocity potential.

Even without continuing the algebra beyond this point it may be noted that the resulting nonlinear differential equation in фі is not amenable to a simple closed solution and that further restrictions on the variables are required. Since all possible restrictions on the generality of the flow properties have already been made, it is necessary to consider the nature of the component velocities themselves.

Small disturbances

So far it has been tacitly assumed that the flow is steady at infinity, and the local flow velocity has components и and v parallel to coordinate axes x and у respectively, the origin of coordinate axes furnishing the necessary datum. Let the equations now refer to a class of flows in which the velocity changes only slightly from its steady value at infinity and the velocity gradients themselves are small (thin wings at low incidence, etc). Further, identify the x axis with the undisturbed flow direction (see Fig. 6.33). The local velocity components и and v can now be written:

и — Uoo + г/. v = v’

where ti and V are small compared to the undisturbed stream velocity, and are termed the perturbation or disturbance velocities. These may be expressed non­dimensionally in the form

Similarly, дііjdx, dV/dy are small.

Making this substitution, Eqn (6.115) becomes

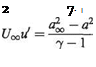(f/3C+t/)2 + /2 | a1 _ |

When the squares of small quantities are neglected this equation simplifies to

similarly

я2 = aL ~ (7- )U^

Also, 1 — (У/Uoo)2— 1 from the small disturbance assumption.

Now, if the velocity potential ф is expressed as the sum of a velocity potential due to the flow at infinity plus a velocity potential due to the disturbance, i. e. Ф = Фоо + Ф, Eqn (6.114b) becomes, with slight re-arrangement:

(1-0H+S Ml

(6.116)

where ф is the disturbance potential and 1/ = дф/дх, V = дф/ду, etc.

The right-hand side of Eqn (6.116) vanishes when М» = 0 and the coefficient of the first term becomes unity, so that the equation reduces to the Laplace equation, i. e. when Mac = 0, Eqn (6.116) becomes

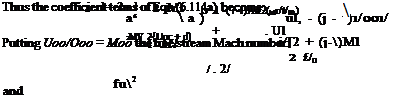
&Ф і &Ф-Г) дх2 dy2

Since velocity components and their gradients are of the same small order their products can be neglected and the bracketed terms on the right-hand side of Eqn (6.116) will be negligibly small. This will control the magnitude of the right – hand side, which can therefore be assumed essentially zero unless the remaining quantity outside the bracket becomes large or indeterminate. This will occur when

M2

l-(7-l )M2ojr

L’OO

i. e. when 7 — l)i/jUoo —> 1. It can be seen that by assigning reasonable values to u’/Ux and 7 the equality will be made when Mx — 5, i. e. put rfjUoo = 0.1, 7 = 1.4, then — 25.

Within the limitations above the equation of motion reduces to the linear equation

<>-<>0+0-

A further limitation in application of Eqn (6.116) occurs when Мж has a value in the vicinity of unity, i. e. where the flow regime may be described as transonic. Inspection of Eqn (6.116) will also show a fundamental change in form as Mx approaches and passes unity, i. e. the quantity (1 — M^) changes sign, the equation changing from an elliptic to a hyperbolic type.

As a consequence of these restrictions the further application of the equations finds its most use in the high subsonic region where 0.4 < M00 < 0.8, and in the supersonic region where 1.2 < Mx < 5.

To extend theoretical investigation to transonic or hypersonic Mach numbers requires further development of the equations that is not considered here.

Prandtl-Glauert rule – the application of linearized theories of subsonic flow

Consider the equation (6.118) in the subsonic two-dimensional form:

(1_M-)0 + 0 = ° W6-118))

For a given Mach number M00 this equation can be written

*0+0- <««>

where В is a constant. This bears a superficial resemblance to the Laplace equation:

д2Ф &Ф W+ dr?~0

and if the problem expressed by Eqn (6.118), that of finding ф for the subsonic compressible flow round a thin aerofoil, say, could be transformed into an equation such as (6.120), its solution would be possible by standard methods.

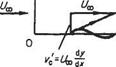
Figure 6.34 shows the thin aerofoil occupying, because it is thin, in the definitive sense, the Ox axis in the real or compressible plane, where the velocity potential ф exists in the region defined by the xy ordinates. The corresponding aerofoil in the Laplace or incompressible £77 plane has a velocity potential Ф. If the simple relations:

Ф = Аф, £ = Cx and 77 = Dy (6.121)

are assumed, where A, C and D are constants, the transformation can proceed.

The boundary conditions on the aerofoil surface demand that the flow be locally tangential to the surface so that in each plane respectively

Compressible xy plane

‘Laplace’ £75 plane

О

x

Fig. 6.34

and

(6.123)

where the suffices c and і denote the compressible and incompressible planes respec­tively.

Using the simple relationships of Eqn (6.121) gives

дФ _ д{Аф) _Адф &Ф _ А &ф д£ ~д(Сх)~Сдх’ д?~С1дх2

and

дФ_Адф &Ф А &ф

dr/ D dy’ drf – D2 dy2

Thus Eqn (6.120) by substitution and rearrangement of constants becomes

(6.124)

Comparison of Eqns (6.124) and (6.119) shows that a solution to Eqn (6.120) (the left-hand part of Eqn (6.124)) provides a solution to Eqn (6.119) (the right-hand part of Eqn (6.119)) if the bracketed constant can be identified as the B2, i. e. when

(6.125)

Without generalizing further, two simple procedures emerge from Eqn (6.125). These are followed by making C or D unity when D = В от 1/C = В respectively. Since C and D control the spatial distortion in the Laplace plane the two procedures reduce to the distortion of one or the other of the two ordinates.