APPLICATION OF THE IMPROVED ALGORITHM

The improved algorithm of subsection 5.2 is applied. Most of the frequency intervals, which correspond to the 50 points of the initial fre­quency gridding, were eliminated, and the basic algorithm of subsec­tion 5.1 is applied only to 8 intervals. The same result is obtained for Umax – The computations were done in only 474 s (about 8 minutes). This improved solution appears thus especially computationally efficient. Moreover, the result is nearly non conservative.

6.1 A PHYSICAL INTERPRETATION OF THE ti LOWER BOUND

The aim of this subsection is to illustrate the usefulness of a q lower bound. Let (А, В, C, 0) a state-space representation of the transfer mat­rix M(s) in the interconnection structure M(s) – Д. A destabilizing perturbation Д* is provided with the fi lower bound. Remember that the value of this lower bound is 1.94 at 9.97 rad/s with the method by (Magni and Doll, 1997). This means that the norm of Д* is 1/1.94 and that the closed loop state matrix A + BA*Chas a pole on the imaginary axis at ± 9.97 rad/s.

This is confirmed by the root locus of Figure 10.5. This root locus
was obtained by plotting the eigenvalues of A + BaA*Cwhen a varies between 0 and 1. The star "*" represents the initial poles (associated to a = 0), while the circle "o" represents the final poles (associated to a = 1). The closed loop rigid poles at the bottom of the Figure are not especially moved by the application of the destabilizing perturbation Д*.

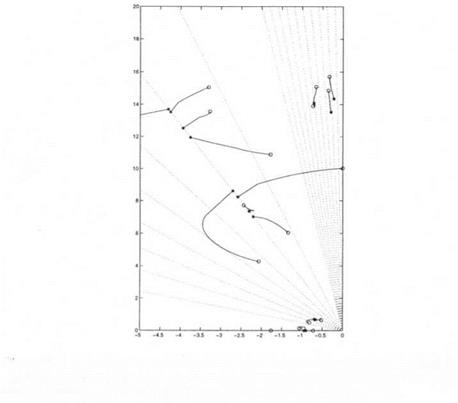Root locus associated to the destabilizing model perturbation, which is

provided with the fi lower bound – the dotted lines each correspond to an isovalue of the damping ratio, namely 1 %, 2 %, … ,10 % and 10 %, 20 %, … ,100 %.

The 2 closed loop flexible modes, which are not actively controlled (see subsection 1.4 of chapter 2), are neither especially moved by the application of A*. Note that two poles are associated to each flexible mode in the figure: one for the state-feedback controller and one for the observer. These two poles do not coincide, even if they are close, because the state-feedback and observer gains were computed with two different methods.

The 4 closed loop flexible modes, which are actively controlled, are

moved indeed by the application of Д*. The closed loop flexible pole, which crosses the imaginary axis, corresponds to the state-feedback closed loop pole:

frequency (rad/s)

-2.59Є+00 + 8.23e+00i 3.00e-01 8.62e+00

-2.59e+00 – 8.23e+00i 3.00e-01 8.62e+00

and thus to the open loop flexible mode:

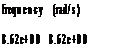damping

-4.39Є-01 + 8.6ІЄ+00І 5.09Є-02

-4.39e-01 – 8.61e+00i 5.09e-02

This suggests that the robustness of the observed state-feedback control­ler could be increased by improving the robustness with respect to an uncertainty in the characteristics of this critical bending mode.