Three-Dimensional Point Source at the Origin of Coordinates

The point source referred to herein is a true point source in that flow exits from a point radially in all directions in three-space. The point source previously discussed in two-dimensional flow (see Chapter 5) is actually the cross section of a line source of infinite length that is perpendicular to the two-dimensional plane under study. Recall that source flow also is radial in two-dimensional, planar flow.

Consider a point source at the origin of a three-dimensional, Cartesian – coordinate system, as shown in Fig. 7.5.

Figure 7.5. Source flow at the origin.

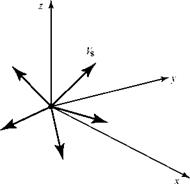By definition, the flow is purely radial, with a velocity given by Vs. We consider a fixed spherical control volume surrounding the origin of coordinates, with the center of the sphere at the origin (Fig. 7.6). Then, we make a calculation for the mass flux through the surface of the control volume. Recall that the surface area of a sphere of radius a is given by 4na2.

We let Л represent the volume flow out of the point source per unit time. Because the source flow is purely radial, the outflow velocity vector is always perpendicular to the spherical control surface and has a constant magnitude over the surface. Thus, the mass flow rate out of the source is:

m = рЛ = pjjV. ndS = pVs jjdS = pVs (4na2). (7.24)

Solving for Vs gives:

V = —Л-.

s 4na2

Contrast this result with the result previously obtained for a two-dimensional point source (i. e., corresponding to the radial flow through a cylindrical control volume with a line source along the axis of the cylinder). In the two-dimensional case, the radial-source velocity varies inversely as the radial distance from the source; in

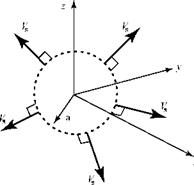Figure 7.6. Mass flux through fixed control volume.

the three-dimensional case, the radial-source velocity varies inversely as the square of the radial distance from the source.