Displacement Thickness

Displacement thickness S* may be defined as the distance by which the boundary would have to be displaced if the entire flow field were imagined to be frictionless and the same mass flow is maintained at any section.

Consider unit width in the flow over an infinite flat plate at zero angle of incidence, and let the x – component of velocity to be Vx and the у-component of velocity be Vy. The volume flow rate Aq through this boundary layer segment of unit width is given by:

Aq = (Vm – Vx) dy,

0

where Vm is the main stream (frictionless flow) velocity component and Vx is the actual local velocity component. To maintain the same volume flow rate q for the frictionless case, as in the actual case, the boundary must be shifted out by a distance S* so as to cut off the amount Aq of volume flow rate. Thus:

•TO

(2.24)

The displacement thickness is illustrated in Figure 2.7. The main idea of this postulation is to permit the use of a displaced body in place of the actual body such that the frictionless mass flow around the displaced body is the same as the actual mass flow around the real body. The displacement thickness concept is made use of in the design of wind tunnels, air intakes for jet engines, and so on.

The momentum thickness в and energy thickness Se are other (thickness) measures pertaining to bound­ary layer. They are defined mathematically as follows:

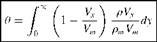(2.25)

_L

b

x

Hypothetical flow with displaced boundary

Figure 2.7 Illustration of displacement thickness.

where Vm and pm are the velocity and density at the edge of the boundary layer, respectively, and Vx and p are the velocity and density at any y location normal to the body surface, respectively. In addition to boundary layer thickness, displacement thickness, momentum thickness and energy thickness, we can define the transition point and separation point also with the help of boundary layer.

A closer look at the essence of the displacement, momentum and energy thicknesses of a boundary layer will be of immense value from an application point of view. First of all, S*, в and Se are all length parame­ters, in the direction normal to the surface over which the boundary layer prevails. Physically, they account for the defect in mass flow rate, momentum and kinetic energy, caused by the viscous effect. In other words:

• The displacement thickness is the distance by which the boundary, over which the boundary layer prevails, has to be hypothetically shifted, so that the mass flow rate of the actual flow through distance S and the ideal (inviscid) flow through distance (S — S*), illustrated in Figure 2.7, will be the same.

• The momentum thickness is the distance by which the boundary, over which the boundary layer prevails, has to be hypothetically shifted, so that the momentum associated with the mass passing through the actual thickness (distance) S and the hypothetical thickness (S — в) will be the same.

• The energy thickness is the distance by which the boundary, over which the boundary layer prevails, has to be hypothetically shifted, so that the kinetic energy of the flow passing through the actual thickness (distance) S and the hypothetical thickness (S — Se) will be the same.