Superposition of Uniform Flow and a Source Flow

Similar to two-dimensional, planar flow (see Chapter 4), it may be shown by sub­stitution that the stream functions described by Eqs. 7.27 and 7.34 are both solu­tions of the defining equation for the stream function in axisymmetric flow, Eq. 7.23. Furthermore, because Eq. 7.23 is a linear equation, the sum of these two solu­tions is also a solution. In particular, the streamline у = 0 is the body surface with a stagnation point. Thus, a valid stream function for axisymmetric flow is given by:

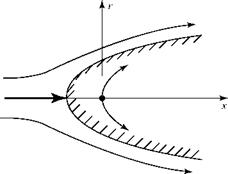
Taking an arbitrary but fixed value of the source strength, Л, and the freestream velocity, VTC, the resulting streamlines may be found by taking various values of у = constant and finding pairs of points (x, r) that satisfy Eq. 7.35. The result is shown in Fig. 7.9.

The flow field is seen to represent the axisymmetric flow over an open – ended body of revolution at zero angle of attack. Figure 7.9 appears the same for any (x, r) plane; thus, for any angle 0 in cylindrical coordinates. Notice that the stagnation point is upstream of the origin of coordinates, as expected. The fineness of the body of revolution may be altered by changing the values of Л and/or VTC.

Figure 7.9. Superposition of a three­dimensional source and uniform flow.

The velocity components ux and ur at any point in the flow field may be evaluated by using Eq. 7.35 in Eq. 7.20.

7.2 Flow Past a Rankine Body

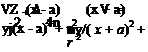The superposition in Section 7.8 may be extended by combining a uni­form axial flow with a three-dimensional source and sink of equal strengths and located a distance, a, upstream and downstream of the origin, respec­tively. For this axisymmetric flow, the equation of the streamlines is given in Karamcheti, 1967 as:

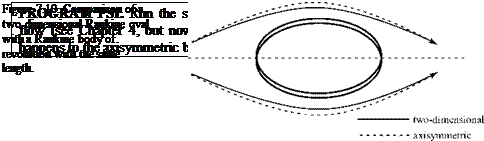The resulting body shape in axisymmetric flow is a body of revolution called a Rankine body. The two-dimensional, planar-flow counterpart of this body is the Rankine oval. The shape of the two bodies and the streamlines associated with them are illustrated in Fig. 7.10.