Aerodynamics of Aircraft in Maneuver

This chapter will examine the aerodynamics of thin wings of arbitrary planform and of slender bodies in arbitrary translation and rotation. Quasi-steady flow will be assumed.

6.1 Aircraft Motion Definition

Chapter 9 will derive in detail the Earth and body axis systems used for describing aircraft motion. Here, a few of those key relations will be simply stated without derivation. Unless otherwise indicated, all vector components will be assumed to be in the geometry axes shown in Figure 6.1, which have x and z reversed from the body axes given in Chapter 9. The other axis systems will be discussed where appropriate.

6.1.1 Aircraft velocity and rotation

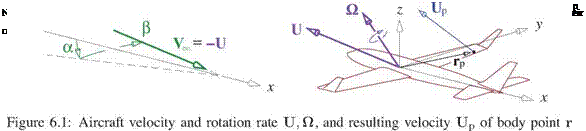The aircraft motion is defined by the velocity U of its axis-origin point, and by its rotation rate П. Both are shown in Figure 6.1. These are defined relative to the Earth frame, and hence they are also the velocity and rotation rate of the aircraft relative to a still airmass.

The aerodynamic “freestream” velocity V, is directly opposite to U, and is conventionally specified by the two aerodynamic flow angles a and в, applied in that order as shown in Figure 6.1.

Ux 1 ( — cos a cos в 1

Uy = —V, = V, I sin в > (6.1)

Uz — sin a cos в

Ко = /ux + Ц? + U.’} , a = arctan—K – , f3 = arct. an — ^4 (6.2)

V ‘T y ~ ’ – Ux ’ ^

Given these reciprocal relations, {К,, а, в} and {Ux, Uy, Uz} are equivalent alternative parameter sets. In practice, а, в are chosen as the independent parameters. These define the three components of the normal­ized aircraft velocity U/V, via (6.1), which are needed to compute the aerodynamic forces and moments.

6.1.2 Body-point velocity

The Earth-frame velocity of any point rp fixed on the body is given by

Up = U + Oxrp (6.3)

as shown in Figure 6.1. If the airmass is still (without wind or gusts), then the apparent airmass velocity seen by this point is —Up, which is in effect a “local freestream.” This will be used to formulate flow-tangency boundary conditions in computational methods.