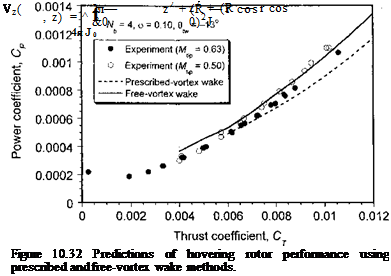
Prescribed Vortex Wake Models for Forward Flight Vortex Ring Model

One simple way to approximate the trailed wake vorticity from the rotor is to used a series of stacked vortex rings or a vortex tube – see Coleman et al. (1945), Castles & De Leeuw (1954), and Young (1974). The advantage of using vortex rings is that an exact (analytic) solution for the induced velocity can be obtained. In the vortex ring (tube) model, one ring represents the trailed wake system generated by one blade during one rotor revolution. The positioning of the rings is defined on the basis of simple momentum theory.

The induced velocity from one disingularized vortex ring of strength

R(R — r cos в) dO

(R2 — 2Rr cos 0 + z2 + r2)3/2

[see, for example, Lewis (1991)]. This equation can be integrated analytically using elliptic integrals of the first and second type. Alternatively, it can be integrated using Gaussian quadrature (see Question 10.1). Notice that for points not on the vortex segment itself, the cut-off distance, 80, equals zero. However, for points on the ring vortex a logarithmic singularity occurs, and so 80 must be nonzero. Although the value of 80 can be approximated in terms of the vortex core radius and an exponential function of the kinetic energy in the vortex core [see Widnall (1972)] in a practical sense a cut-off distance of 80 ~ 10 ~4 can be assumed without substantial error.

From this basic result for one ring, the net induced velocity at any point in the flow field can be obtained by summing the effects of all the rings representing the wake. It has been found from the vortex ring (tube) model that the longitudinal distribution of inflow in the rotor TPP is approximately linear, which is in good agreement with experiments – see Coleman et ai. (1945). Also, it has been found that the longitudinal coefficient kx m the linear inflow model can be approximated using kx = tan(x/2), which is a result discussed previously in Section 3.5.2.