PROPERTIES AND DESIGN OF SLENDER AIRCRAFT. FOR SUPERSONIC FLIGHT

6.1 The evolution of the design concept. The aerodynamic design concept of slender wings differs radically from that of the classical and swept-winged types of aircraft, which has been described so far. We want to consider now how this new design concept may be applied to flight at supersonic speeds.

How the design concept evolved from some basic considerations of fluid mecha­nics is not without some instructive value in itself and will be discussed first.

When the possibility of supersonic aivil flight was seriously considered in the early fifties, a great deal was known about the aerodynamics of bodies of revolution and of twodimensional aerofoils as well as unswept wings of finite span. Applying the classical design principles and using the numbers associa­ted with this set of aerodynamics for the various factors involved, the results turned out to be most disappointing. It seemed inevitable that, on the aerodynamics alone, supersonic long-range flight would be uneconomic if not altogether impossible. Therefore, some new piece of information had to be fed into the argument if we were to proceed at all. This came from the propulsion side in that it became clear that, for the same physical reason that makes the aerodynamics of wings and bodies deteriorate at supersonic Mach numbers, the aerodynamics of jet engines improves, so that we find a steady increase of the propulsive efficiency rip with Mach number, within limits. This was probably first realised by К Oswatitsch (1944, unpublished) and applied to ramjet propulsion, but it applies also to turbojets. To put some numbers to this, we may write typically:

which was suggested by R 6 Thorne (1956, unpublished) and has been used before in section 1.2 as a rough guide. The question then arises of whether this improvement in propulsive efficiency can compensate for the drag increase and the corresponding deterioration of the lift-to-drag ratio, L/D.

With this in mind, studies of conventional wing-fuselage layouts were under­taken. To give an example, one of these arrived at an unswept wing of aspect ratio 2 and of only 3|% thickness-to-chord ratio on a very slim fuselage of fineness ratio 1:20 and a high wing loading of 8.4kN/m^, the total mass at take-off being about 1.6 x lO^kg. It was concluded that it might just be possible to operate this type of airliner at Mq = 2 nonstop between London and New York with about 18 passengers, but that the economic fare might be some three times that of those days and about five times that of the high – subsonic jet aircraft which were then being considered. Note that the pay­load fraction of this supersonic aircraft would be about 1%. The answer, therefore, was that the expected improvements in the propulsive efficiency did not make up for the aerodynamic deficiencies of this type of aircraft.

Yet another input of new information was, therefore, needed if supersonic flight was to be economic. Results for a new set of aerodynamics became
available during the 1940s and early 50s which showed that pointed delta wings of small aspect ratio could have lower wavedrags at supersonic speeds than unswept wings (see sections 6.7 and 6.8 below). To our knowledge, the first attempt to exploit these properties and to use narrow delta wings in practical aircraft design was made by A A Griffith (1954, unpublished). This was in some military application but J R Collingbourne (1955, unpublished) pointed out soon afterwards that the concept was more promising as a civil long-range aircraft. In these studies, very low values of the aspect ratio, below 0.5, were considered and it was assumed as a matter of course that such aircraft would not be able to take-off or land unassisted. Griffith, there­fore, proposed to instal a large number of small jet engines within the wing and to use these to produce direct jet lift at low speeds. This introduced so many aerodynamic, structural and other complications that the attempt was abandoned.

Thus it was in 1955 that it was recognised clearly, for the first time, that Cayley’s design principle could not be applied to reach the objective of economic supersonic flight; that а new set of aerodynamic design principles would have to be thought out to reach this objective.

Bearing in mind the stature of Cayley’s reasoning and imagination, it was also clear that any new design principles, to stand side-by-side with Cayley’s, would have to prove that new shapes and layouts, associated with a new type of flow, would be as effective in engineering applications for the new objec­tive as the classical principles had been for their objectives. Thus the flow should again have the same basic physical features as the classical aerofoil flow: it should be steady, stable and controllable, changing quantitatively with changes in attitude and Mach number while remaining qualitatively of the same type throughout the whole flight range. As it turned out, the shape and flew of the slender wing could fulfil these conditions and thus a new design concept emerged, that could stand side-by-side with that of the classical aircraft, in its own right.

It took two essential steps to evolve the new aerodynamic design concept of the slender wing. In the first step (D KUchemann (1955)), the concept of controlled flow separation was introduced, based on the first precise defini­tion of what we mean by flow separation in three dimensions, which had only then been given by E C Maskell (1955), and on an analysis of the effects of flow separations, from existing experimental data, by J Weber (1955). The application of controlled separation in aerodynamic design was then explained in detail by E C Maskell & D KUchemann (1956), and the new design principles were summarised later by E C Maskell & J Weber (1959) and by E C Maskell (1961). Actually, it began with the realisation that the threedimensional flow patterns near a rounded and swept leading-edge would be like those sketched in Fig. 2.5, with the possibility that the limiting streamlines in the surface could readily run into an envelope and form an ordinary separation line. This led to serious doubts about whether it would be reasonable to attempt to keep the flow attached as in the classical aerofoil type of flow when the angle of sweep was high. This then provoked the question of what types of flow could be expected if the flow was allowed to separate? Simple reasoning then led to the flow patterns sketched in Fig. 2.8, and it became clear immediately that the wholly-mainflow pattern, without bubbles but with vortex sheets springing from fixed primary lines of separation, should be the preferred engineering solution. To fulfil the condition that the type of flow should remain the same throughout the whole flight range, the separation lines must be kept in the same place, i. e. they must be fixed along salient

and aerodynamically sharp edges. This led to the conclusion that the sharp edge was a much more valuable means of controlling the flow than had hitherto been realised. On the other hand, vortex sheets may spring from any line of separation on a threedimensional lifting body, not only from the trailing edge: it is not obvious that separation from a trailing edge only is prefer­able to any other pattern of separation. Thus separation was considered to be able to play an essentially constructive role, and this led to the question of how wings with flow separation from all edges, including sharp leading edges, might behave. Planforms where both leading and trailing edges are highly swept could be ruled out: the flow pattern, such as that sketched in Fig. 4.37, has too many undesirable features. Thus the natural outcome of the preceding arguments was a planform with highly-swept and sharp leading (and, possibly, side) edges and a nearly unswept and sharp trailing edge, i. e. some variant of the highly-swept, slender, sharp-edged delta wing.

The flow is then of the type sketched in Fig. 3.6. It is possible to maintain it at subsonic speeds and also at supersonic speeds. To ensure that the coiled vortex sheets lie always on the same side of the wing (upper or lower), and spring from the whole length of each edge, the sharp leading edges must be attachment lines at one particular attitude and speed: this can be chosen to occur at or near the cruising condition. Among the merits of this new type of aircraft with its new type of flow, which could readily be foreseen at the time, where the beneficial characteristics at low speeds, providing enough lift without any aid from engines (to be discussed in more detail in section 6.5 below) and also the chance of achieving a fully-integrated design, where the means of providing volume and lift are combined in one wing: a separate fuselage should be quite unnecessary from the aerodynamic point of view. Further, trailing-edge flaps and a fin should be sufficient for control pur­poses: there is no need for a tailplane.

After this, a second step had to be taken to complete the evolution of the aerodynamic design concept of the slender wing, namely, to show that the prin­ciples described so far could lead to a practical aircraft that could perform the desired task of crossing the Atlantic, say, economically and at some supersonic speed; and that there need be no fundamental conflicts between the requirements for low-speed flight and high-speed flight. This second step was taken soon afterwards (D KUchemann (1957)); see also D Kllchemann (1962)). It was already known at the time that some constraints had to be imposed on the planform shape to achieve the required lift at low speeds at a sufficiently low angle of incidence and with acceptable flying characteristics. Very roughly, all these conditions led to a constraint on the slenderness ratio that could be admitted: the semispan-to-length ratio, s/% , should be around 0.25; it should not be appreciably lower than about 0.2 and values above 0.5, say, would imply somewhat low angles of leading-edge sweep and would make the application of the flow concept rather pointless.

Another constraint is imposed by the volume that must be provided: for a transport aircraft of medium size, the volume coefficient т, defined by equation (4.139), should have a value around 0.04. Next, the question had to be considered of whether the required long-range performance could be achieved with a wing of this slenderness ratio and volume. On the assumption that an aircraft could be built, having a reasonable payload and being nearly half­full of fuel at take-off like subsonic aircraft, it follows that the product HpL/D should be about 3, according to Brdguet’s range relation. Hence a typical target for the aerodynamic efficiency was defined by R G Thorne (unpublished) as

using the approximation of equation (6.1). Now a drag relation like (4.140) could be applied and sets of values of т and s/& , which satisfied (6.2), could be calculated for various flight Mach numbers using some typical values for the drag factors involved: Ко – 1» Kv = = 1.2, Сщр = 0.004, p – J

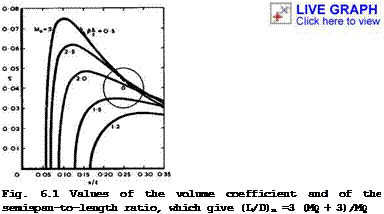(these matters will be set out in more detail in section 6.2 below). The results are shown in Fig. 6.1 and establish an obvious ’ballpark1. They

demonstrated at that early stage that the required aerodynamic performance should indeed be achievable with the volume needed and with slenderness ratios wanted for low^speed flight. It was also recognised that the aerodynamic slenderness ratio $sIl should be near 0.5, which implied subsonic leading edges and confirmed that the desired type of flow could be realised at all speeds. The results also showed that a flight Mach number near 2 would be a suitable target for this type of aircraft: lower cruising speeds should be left to swept wings (see section 4.9); to aim for substantially higher cruis­ing speeds did not appear to offer worthwhile returns. Flying near Mq * 2 would reduce the flying time* drastically, compared with that of subsonic aircraft, and would, at the same time, avoid severe aerodynamic heating and so allow light-alloy construction to be used, thus avoiding the complications which result from the application of other materials of construction.

Thus this second step completed the general case for this new type of aircraft and established the practicality of the design concept and, in particular, the

* What matters to the operator is the number of return trips per day an air­craft can make (between London and New York, say), which is a measure of its ■productivity. On the assumption that the turnround time is 2,5 hours and that no one wishes to arrive or depart between midnight and eight o’clock in the morning, timetables can be worked out, which show that a subsonic air­craft will manage one daily return trip whereas a supersonic aircraft can do two if it flies at a Mach number above about 1.8, i. e. the supersonic air­craft doubles the productivity. Another important point is that no further increase in the number of trips is possible as the Mach number increases above about 1.8 until it approaches 3. Thus a cruising Mach number near 2 represents a significant step for the operator.

essential compatibility between the low-speed and the supersonic character­istics of slender wings. In short, everything fits together. It may be worth drawing attention to the fact that the new type of aircraft did not result from an investigation of a systematic series of wing geometries or from any optimisation procedure: It was the outcome of reasoning in terms of funda­

mental fluid mechanics.

As soon as the general design concept was established, a large number of problems to be solved could be identified. This gave impetus to a large – scale research exercise coordinated by the Supersonic Transport Aircraft Committee (STAC) during 1956 to 1959. Results will be discussed below. Here, we refer to some more general papers recording these matters by M J Lighthill

(I960), M В Morgan (1960) and (1971), D KUchemann (1960) and (1962) A Spence & J H В Smith (1962), A Spence & D Lean (1962), L F Nicholson (1962),

R L Maltby (1968), and D KUchemann & J Weber (1968),