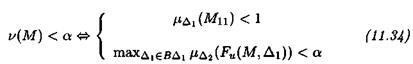COMPUTATIONAL METHOD

The following Lemma is introduced as a preliminary. It can be proved in the same way as Lemma 2..1 of chapter 8.

LEMMA 3..1 Let M a complex matrix, Ді a mixed model perturbation which is to be maintained inside its unit ball, and Д2 a mixed model perturbation whose size is free. Then:

We now come back to the initial problem. Remember that Д 1 is a mixed model perturbation which gathers the parametric uncertainties and neg­lected dynamics, while Д2 = diag(8{,… ,6^) is a structured complex model perturbation corresponding to time delays. The associated inter­connection structure M – Д, with Д = diag(AьДг), is presented in Figure 11.8. Note that matrices M and Д are the values of the transfer matrices M(s) and Д(й) at s = ju. Frequency to is fixed in the following.

The idea is simply to use the first v upper bound of chapter 8 in order to compute a lower bound of the robust delay margin. A is to be main­tained inside its unit ball, while the size of Д2 is free. The difficulty is to prove that a lower bound of the robust delay margin is indeed obtained with this approach.

Let mi the dimension of Д*. In the same way as in chapter 8 (section L), scaling matrices D_{ associated to perturbations A{ are introduced (*.e DiAi = AiDi)- D and £>2 are then defined as:

so that D = D + D2 is a scaling matrix associated to Д. a is an upper bound of і’д(М) if the following LMI is satisfied:

When multiplying the above inequality on the left and on the right by:

The above LMI becomes: XMfDi + Di)MX – XDX + j(XGMX — XM’GX) < a2P2 (11.38)

Let:

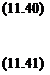
Di = XDiX M = X~lMX G = XGX

Equation (11.38) can be rewritten as:

M*{DX + P2)M – Di+ j(GM – M*G) < a2P2

The key point is to note that the above LMI implies that a is also an upper bound of і’д(М), with:

A = diag(A ЬД2) (11.42)

and A2 is a full complex block with the same dimensions as A2.

As a consequence, using Lemma 3..1 and the fact that 2(H) = o(H) for any complex matrix H, it can be claimed that:

Noting that FU{M, A) = D^2Fu(M, Ai)D21^2, the above property is rewritten as:

(t(D2^2Fu(M, A)D2 l^2) < a (11.44)

Remember that FU{M, A{) is the transfer matrix seen by the complex structured perturbation A2, which models the time delays (see Fig­ure 11.8). Following subsections 3.1 and 3.2, the above equation means that the sufficient condition of robust stability, with respect to uncertain time delays, is satisfied for all Ai Є BA 1. a can thus be used in equa­tions (11.25) and (11.27) to compute lower bounds of the robust MIMO phase and delay margins.