INTRODUCTION OF MODEL UNCERTAINTIES

3.3.1 INTRODUCTION

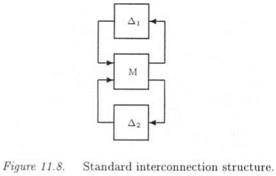
Consider the general case of a standard interconnection structure M(s) – A(s),with A(s) = diag(Ai (s), A2(s)): Ai(s)is a mixed model perturb­ation gathering all classical model uncertainties, whereas Дг(з) gathers all uncertain time delays (see equation (11.4)). As proved in the follow­ing, the issue of computing a lower bound of the robust delay margin essentially reduces to a skewed ц problem, in which the mixed model perturbation Ді is to be maintained inside its unit ball, while the size of the model perturbation Д2 is free.

The problem is however more complex than it may appear at a first glance: when the only uncertainties in the closed loop are the time delays, it was indeed remarked in the previous subsections that the small gain approach provides a conservative value of the MIMO delay margin, even if the exact value of the s. s.v. is computed. In other words, the small gain theorem, with or without scaling matrices, provides just a sufficient condition of stability. In the same way, even if the problem of computing a lower bound of the robust delay margin essentially reduces to a skewed H problem, this does not mean that the exact value of the robust delay margin would be obtained if the exact value of the skewed s. s.v. could be computed.