Lifting Surface Theory

Lifting surface theory is an extension of thin airfoil theory to 3D. It models the flow about the wings and tails of a general 3D aircraft configuration using vortex sheets y(s,£), or the equivalent normal-doublet sheets p(s, e). The objective is to represent the lift, sideforce, moments, and induced drag of the configuration using only the camber surface shapes, with the volume effects of the various components being ignored.

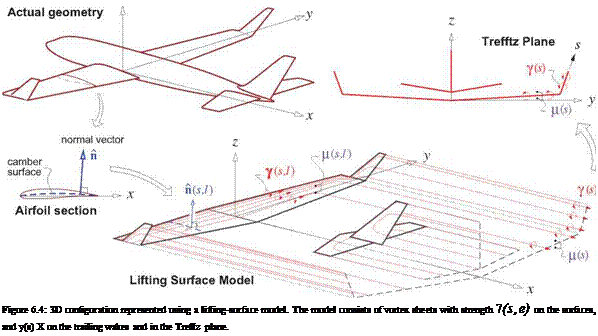
6.4.1 Vortex/doublet sheet geometry

The assumed geometry of the vortex or doublet sheets is shown in Figure 6.4. The sheets are assumed to be everywhere parallel to the x axis, with the camber-surface shapes of the actual geometry represented only by their normal vector distribution n(s, e). The sheet strengths 7(s, e) or p(s, e) are unknown only over the extent of the actual surface. On the trailing wake portions of the sheets, the strengths are constant in x, and equal to their trailing-edge values.

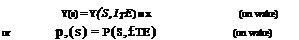(6.16)

These are also the sheet strengths in the Trefftz plane, as shown in Figure 6.4.

6.4.2 Lifting-surface problem formulation

The perturbation velocity field of the vortex sheet distribution is given by the superposition integral (2.16),

where r'(s,£) is the assumed vortex sheet geometry. The integral is over both the surface and wake vortex sheets. With an airmass which is still in the Earth frame, the total fluid velocity observed by a point r fixed in the body frame is then obtained by subtracting that point’s velocity Up as given by (6.3).

V(r) = V7 – (U + Oxr) (6.18)

The flow-tangency boundary condition is then

for each r(s, e) surface point. The Kutta condition

Y(s, eTE) x X = 0 (6.20)

is also applied all along the trailing edge of each surface. Equations (6.17), (6.19), (6.20) together constitute an integral-equation problem for the unknown 7(s, e) distribution. If instead p(s, e) is chosen as the unknown variable, then 7 = П x Vp would be substituted into all the above expressions. This lifting-surface problem can be solved by the Vortex Lattice Method, described later in Section 6.5.