Newton’s Impact Theory

The amount of pressure exerted on a surface with impinging flow is equal to the normal component of the momentum exerted by the impinging fluid particles. The theory proposed by Newton and based on this impact idea was interpreted, until the beginning of twentieth century, as the cause of the aerodynamic force acting on flying objects! The impact theory on the other hand was not able to produce sufficient lifting force to balance the weight of flying creatures in nature. Naturally, during the years of the emergence of the impact theory and for the following couple of centuries to come the relation between the air speed and the compressibility of air was not known. Therefore, by shear coincidence the eval­uation of surface pressure coefficient with the impact theory, independently from the Mach number itself, at very high Mach numbers was given in the first half of the twentieth century (Hayes and Probstein 1966). Now, let us evaluate the pressure exerted by the impact theory on a wall inclined with angle 0w in a freestream speed of U. The amount of mass per unit time per unit area of the air particles striking the wall, shown in Fig. 7.1, is pUn. The momentum exerted on the wall by this air mass is p(Un)2 which is also equal to the pressure exerted.

Expressing the wall pressure in terms of the free stream speed we obtain

p = pU2 sin2 Qw (7.1)

If the area of the wall shown in Fig. 7.1 is S, and the region behind the wall is considered as a vacuum, the normal force acting on the wall becomes

N = pS = SpU2 sin2 Qw (7.2)

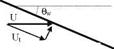Fig. 7.1 Velocity compo­nents of the fluid particles impinging on a wall at a free stream speed of U

The normal force coefficient then reads

From this normal force coefficient we can obtain the lift coefficient normal to the free stream and the drag coefficient in the direction of free stream as follows

Cl = 2 sin2 0w cos hw and Cd = 2 sin3 hw (7.4a, b)

Since the wall angle and the angle of attack is the same for a flat plate, according to the impact theory at small angles of attack the lift coefficient is proportional with the square of the angle of attack, Eq. 7.4a, b. This lift coefficient is not sufficient even at considerably high speeds (This was the excuse to explain ‘flying is a property of heavenly bodies’ for centuries). We can now express the surface pressure coefficient, using the normal force coefficient given by Eq. 7.3, in terms of the free stream Mach number

, P – Pi _ P Pi _ 2 2 h _ _A_

‘p 1/2pU2 1/2pU2 1/2pU2 w yM2

In hypersonic flow the free stream Mach number is high and its square is very high. Therefore, the second term of the right hand side of Eq. 7.5 is negligible compared to the first term. Neglecting the second term gives us the approximate expression which is independent of free stream Mach number as follows:

Cp ffi 2 sin2 0w