Solutions to Problems
15.1 Solution to Problem 1
15.1.1 Thin Airfoil Theory (2-D Inviscid Flow)
15.1.1.1 Quiz
In subsonic flow, the aerodynamic coefficient dependency upon the small parameters is given in Table 15.1. In supersonic flow, the aerodynamic coefficient dependency upon the small parameters is given in Table 15.2.
15.1.1.2 Lift Coefficient
The lift coefficient Ci in incompressible flow (low speed) is given by
|
|
At M0 — 0.7, using Prandtl-Glauert correction one finds
15.1.1.3 Drag Coefficient
If the drag coefficient of the same profile at a — 0 and M0 — 1.4 is (Cd)M0—1.4 — 0.02, the drag at M0 — 2. is given by © Springer Science+Business Media Dordrecht 2015
J. J. Chattot and M. M. Hafez, Theoretical and Applied Aerodynamics,
DOI 10.1007/978-94-017-9825-9_15
|
Aerodynamic coefficient |
e/c |
d/c |
a |
|
Ci |
n |
y |
y |
|
Cm, o |
n |
y |
y |
|
C m, ac |
n |
y |
n |
|
Cd |
n |
n |
n |
|
Table 15.1 Subsonic flow |
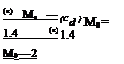 |
|
Aerodynamic coefficient |
e/c |
d/c |
a |
|
Ci |
n |
n |
y |
|
Cm, o |
n |
y |
y |
|
Cm, ac |
n |
y |
n |
|
Cd |
y |
y |
y |
|
Table 15.2 Supersonic flow |
At M0 = 2. if a — 5° then
The drag coefficient at M0 = 0.7 is
(Cd )M0=0.7 = 0
since this is a 2-D, inviscid, subsonic flow.











