Our heavyweight helicopter equal in the world does not have
In Rostov started production of the most load-lifting rotary-wing car The Russian holding «Helicopt[...]
Boundary-Layer Thickness
 |
 |
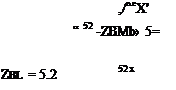The boundary-layer thickness is defined previously (see Fig. 8.9) as the distance from the wall at which the velocity is “essentially” that of the external stream. The Blasius solution indicates that the boundary-layer velocity profile, u(z), approaches the velocity at the edge of the boundary layer, Ue, asymptotically. The boundary-layer thickness, then, may be defined precisely (but arbitrarily) as the distance from the surface at which the velocity, u, is an agreed-on percentage of the external flow velocity. An often-used definition is f = u/Ue = 0.994 as the edge of the boundary layer (i. e., the velocity, u, in the boundary layer is 99.4 percent of the external freestream velocity, Ue), as shown in Fig. 8.11. Then, from Table 8.1:
 |
 |
In this equation:
and the subscript x on the Re number signifies that it is to be based on a length x from the plate leading edge to the downstream station in question.
Eq. 8.68 provides an explicit expression for the magnitude of the boundary – layer thickness, but it is neither useful nor unique. For instance, if it were decided to define the magnitude of 5 as the value of z where the velocity ratio u/Ue is 0.999 (i. e., u is within 0.1 percent of the external velocity), then Table 8.1 indicates that the numerical factor in Eq. 8.51 is now about 6.01; there is a larger constant in the expression for 5 in Eq. (8.51). However, no matter which value of u/Ue that is chosen to define 5(x), it is always true that the boundary-layer thickness on a flat plate varies inversely as the square root of the Re number. Considering the additional linear dependence on x in the numerator, it can be seen that the thickness grows as the square root of the distance downstream from the leading edge.
Thus far, we have found the velocity profile for the flat-plate boundary layer and how the boundary layer grows. Now we define two other thickness properties of the boundary layer that have certain advantages over 5. In particular, they yield unique values for the thickness in any given situation.
