The k Method

Subsequent to Theodorsen’s analysis of the flutter problem, numerous schemes were devised to extract the roots of the “flutter determinant” and thereby identify the stability boundary. Scanlan and Rosenbaum (1951) presented a brief overview of these techniques as they were offered during the 1940s. It was fairly common to include in the flutter analysis a parameter that simulated the effect of structural damping. Observations at that time indicated that the energy removed per cycle during a simple harmonic oscillation was nearly proportional to the square of the amplitude but independent of the frequency. This behavior can be characterized by a damping force that is proportional to the displacement but in phase with the velocity.

To incorporate this form of structural damping into the analysis of Section 5.3.2, Eqs. (5.54) can be written as

m(h + bxe0) + khh = – L + Dh IP 9 + mbxg h + kgg = M + Dg where the dissipative structural damping terms are

Dh = Dh exp(i at)

= – ighma>2hH exp(i at)

Dg = Dg exp(i at)

= – igg Ipagjg exp(i at)

Proceeding as before, Eqs. (5.62) become

The damping coefficients gh and gg have representative values from 0.01 to 0.05 depending on the structural configuration. Most early analysts who incorporated this type of structural damping model into their flutter analyses specified the coefficient values a priori with the intention of improving the accuracy of their results.

Scanlan and Rosenbaum (1948) suggested that the damping coefficients be treated as unknown together with m. In this instance, the subscripts on g can be removed. Writing a = mh/me as before, and introducing

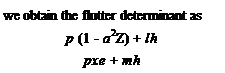Z = © 2(1 + ig) (5.67)

which is a quadratic equation in Z. The two unknowns of this quadratic equation are complex, denoted by

The computational strategy for solving Eq. (5.68) proceeds in a manner similar to the one outlined for Eq. (5.63). The primary difference is that the numerical results consist of two pairs of real numbers, (m1, g1) and (m2, g2), which can be plotted versus airspeed U or a suitably normalized value such as U/(bme) or “reduced velocity” 1/к.

Plots of the damping coefficients g1 and g2 versus airspeed can indicate the margin of stability at conditions near the flutter boundary, where g1 or g2 is equal to zero. These plots proved to be of such significance that the technique of incorporating the unknown structural damping was initially called the “U-g method.” Recalling that the methodology presumes simple harmonic motion throughout, the numerical values of g1 and g2 that are obtained for each к can be interpreted only as the required damping coefficients (of the specified form) to achieve simple harmonic motion at frequencies m1 and m2, respectively. The damping as modeled does not really exist; it was introduced as an artifice to produce the desired motion—truly an artificial structural damping.

The plots of frequency versus airspeed in conjunction with the damping plots can, in many cases, provide an indication of the physical mechanism that leads to the instability. The values of frequency along the U = 0 axis correspond to the coupled modes of the original structural dynamic system. As the airspeed increases, the individual behavior or interaction of these roots can indicate the transfer of energy from one mode to another. Such observations could suggest a way to delay the onset of the instability. To confirm identification of the modes of motion for any specified reduced frequency, it is only necessary to substitute the corresponding eigenvalues, mi and gi, into the homogeneous equations of motion to compute the associated eigenvector (h/b, Є). Because this is a complex number, it can provide the relative magnitude and phase of the original deflections h and e.