Viscous Flows

In the previous sections of this chapter, we have seen many interesting concepts of fluid flow. With this background, let us observe some of the important aspects of fluid flow from a practical or application point of view.

We are familiar with the fact that the viscosity produces shear force which tends to retard the fluid motion. It works against inertia force. The ratio of these two forces governs (dictates) many properties of the flow, and the ratio expressed in the form of a nondimensional parameter is known as the famous Reynolds number, ReL:

where V, p are the velocity and density of the flow, respectively, g is the dynamic viscosity coefficient of the fluid and L is a characteristic dimension. The Reynolds number plays a dominant role in fluid flow analysis. This is one of the fundamental dimensionless parameters which must be matched for similarity considerations in most fluid flow analyses. At high Reynolds numbers, the inertia force is predominant compared to viscous forces. At low Reynolds numbers the viscous effects predominate everywhere, whereas at high Re the viscous effects confine to a thin region, just adjacent to the surface of the object present in the flow, and this thin layer is termed boundary layer. Since the length and velocity scales are chosen according to a particular flow, when comparing the flow properties at two different Reynolds numbers, only flows with geometric similarity should be considered. In other words, flow over a circular cylinder should be compared only with flow past another circular cylinder, whose dimensions can be different but not the shape. Flow in pipes with different velocities and diameters and flow over aerofoils of the same kind are also some geometrically similar flows. From the above-mentioned similarity consideration, we can infer that geometric similarity is a prerequisite for dynamic similarity. That is, dynamically similar flows must be geometrically similar, but the converse need not be true. Only similar flows can be compared, that is, when comparing the effect of viscosity, the changes in flow pattern due to body shape should not interfere with the problem.

For calculating Reynolds number, different velocity and length scales are used. Some popular shapes and their length scales we often encounter in fluid flow studies are given in Table 2.1. In the description of Reynolds number here, the quantities with subscript to are at the freestream and quantities without subscript are the local properties. Reynolds number is basically a similarity parameter. It is used to determine the laminar and turbulent nature of flow. Below a certain Reynolds number the entire flow is laminar and any disturbance introduced into the flow will be dissipated out by viscosity. The limiting Reynolds number below which the entire flow is laminar is termed the lower critical Reynolds number.

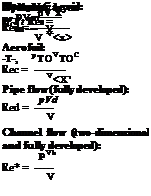Table 2.1 Some popular shapes and their characteristic lengths

Some of the well-known critical Reynolds number are listed below:

Pipe Bow – Red = 2300: based on mean velocity and diameter d.

Channel Bow – Reh = 1000 (two-dimensional): based on height h and mean velocity.

Boundary layer flow – Ree = 350: based on freestream velocity and momentum thickness в.

Circular cylinder – Rew = 200 (turbulent wake): based on wake width w and wake defect.

Flat plate – Rex = 5 x 105: based on length x from the leading edge.

Circular cylinder – Red = 1.66 x 105: based on cylinder diameter d.

It is essential to note that, the transition from laminar to turbulent nature does not take place at a particular Reynolds number but over a range of Reynolds number, because any transition is gradual and not sudden. Therefore, incorporating this aspect, we can define the lower and upper critical Reynolds numbers as follows.

• Lower critical Reynolds number is that Reynolds number below which the entire flow is laminar.

• Upper critical Reynolds number is that Reynolds number above which the entire flow is turbulent.

• Critical Reynolds number is that at which the flow field is a mixture of laminar and turbulent flows.

Note: It is important to note that when the Reynolds number is low due to large viscosity v the flow is termed stratified Bow, for example, flow of tar, honey etc. are stratified flows. When the Reynolds number

Lift force

is low because of low density, the flow is termed rarefied Bow. For instance, flow in space and very high altitudes, in the Earth’s atmosphere, are rarefied flows.