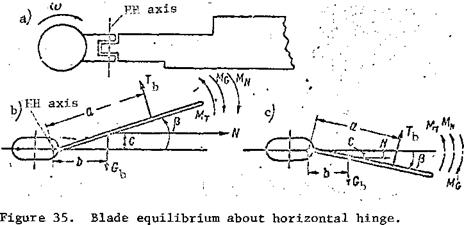
Conditions for Blade Equilibrium Relative. to the Horizontal Hinge

Let us examine the blade thrust moment relative to the horizontal hinge.

If this moment is not transmitted to the hub but simply rotates the blade, then a question arises immediately: how is the blade thrust transmitted

through the hinge to the hub? In order to answer this question we examine

the conditions for blade equilibrium relative to the horizontal hinge.

In addition to the thrust force, in the plane perpendicular to the hub /51

rotation plane there act the weight force G^ and the centrifugal force N (Figure 35b).

Each of these forces develops a moment relative to the horizontal hinge.

The blade thrust moment rotates the blade upward. The flapping angle 3 is formed between the blade longitudinal axis and the hub rotation plane. When the blade tip is above the hub rotation plane, the blade flapping angle is considered positive.

The thrust moment rotates the blade in the direction of increasing flap­ping angle (blade flaps upward). The weight moment = G^b rotates the blade downward, reducing the flapping angle. The centrifugal force moment rotates the blade to bring it closer to the hub rotation plane. If the flapping angle is positive, the centrifugal force moment = Nc rotates the blade downward and coincides in direction with the blade weight force moment. If the flapping angle is negative (Figure 35c), the centrifugal force moment rotates the blade upward and coincides in direction with the thrust force moment. Thus the centrifugal force moment tends to reduce the deflection of the blade from the hub rotation plane.

The centrifugal force always acts in the plane of rotation, is directed outward from the axis, and is applied to the blade center of gravity. It is defined by the formula

gr у Б

The blade centrifugal force of the Mi-4 helicopter at maximal main rotor rpm exceeds 20,000 kgf. Therefore, even with a small arm, c the moment of this force will be very large.

After seeing what moments act on the blade about the horizontal hinge, we can define the equilibrium condition

This condition can he written as follows for positive and negative flap­ping angles:

for g > 0

MT – MQ + MN or Ta – Gb + N – ; <17>

. b

for g < 0

MQ = MT -f – iAft.

Equilibrium in the case of negative flapping angles is possible, hut only in the course of a very limited time. Therefore in the following we shall con­sider (17) to be the equilibrium condition.

If this condition is violated, the blade will rotate until equilibrium is restored at a new flapping angle. With change of the flapping angle, there will be a change of the centrifugal force arm and therefore of its moment. /52

Thus the blade will flap upward if the thrust force moment is greater than the sum of the moments of the centrifugal force and the weight force, i. e., for. But with increase of the flapping angle, the moment = Nc will increase and equilibrium will again be established. The same process will take place upon reduction of the flapping angle, but in the reverse direction.

The flapping angle has a comparatively small value — 7 – 10°.

The primary reason for violation of blade equilibrium relative to the horizontal hinge is the variation of the blade thrust and its moment.

The horizontal hinges have snubbers (stops) to limit the blade upward and downward rotation. The lower stop is the blade droop limiter, i. e., the blade rests on this stop if the rotor is not turning, which prevents the blade coming into contact with other parts of the helicopter. The stop has a centrifugal regulator which allows the blade to deflect to negative flapping angles in flight.

The upper stop limits the upward rotation of the blade (flapping angle 25 – 30°). The blade does not reach the limiters in flight, since the centrifugal force moment does not permit the blade to deflect very far from the hub rotation plane.