Finite-Element or Panel Methods in Quasi-Static Aeroelasticity
Analyzing quasi-static aeroelastic effects requires balancing air loads against structural stiffness and mass distributions. Because of the complexity of the problem, only approximate methods were available for many years. The advent of finite-element or panel methods both in structural analysis and in aerodynamics made accurate quasi-static aero – elastic analysis really possible for the first time.
In the aerodynamic finite-element approach, the airplane’s surface is divided into many generally trapezoidal panels, or finite elements. Under aerodynamic and inertial loadings, the structure finds an equilibrium when boundary conditions are satisfied at control points such as the center of the 3/4-chord line of an aerodynamic panel or at the edges of a structural panel. Finite-element methods in structural analysis preceded those for aerodynamic analysis by many years.
|
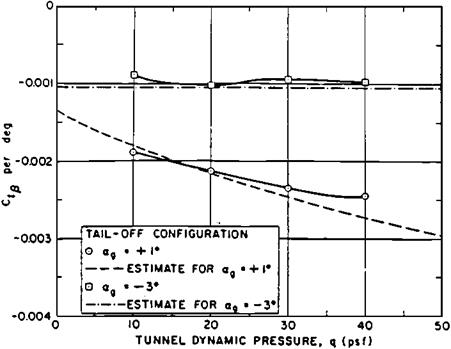
Figure 19.8 Effect of dynamic pressure on the dihedral effect of the Douglas XA3D-1 airplane, at two angles of attack. The wing lift is close to zero at a (fuselage) angle of attack of -3 degrees, and there is little wing bending and change in dihedral effect. (From Rodden, AGARD Report 725, 1989) |
The earliest aerodynamic finite-element method, called vortex lattice analysis, appears to have been developed independently by two people. Vortex lattice analysis is documented in internal Boeing Company and Swedish Aeronautical Research Institute reports by P. E. Rubbert in 1962 and Sven G. Hedman in 1965, respectively, and in a few other reports of the same period. Dr. Arthur R. Dusto and his associates at the Boeing Company combined these structural and aerodynamic finite-element methods into an aero – elastic finite-element system they call FLEXSTAB (Dusto, 1974) in the period 1968 to 1974.
Finite-element methods in quasi-static aeroelasticity require generation of mass, structural influence, and aerodynamic influence matrices. The mass matrix is the airframe mass assigned to each element. The structural influence coefficient matrix transforms deflections at control points in an element to elastic forces and moments at the other elements. The aerodynamic influence coefficient matrix transforms angle of attack at one element to aerodynamic forces and moments acting on the other elements.
It is interesting that the advent of finite-element quasi-static aeroelastic methods coincided with the need for methods that account for significant chordwise structural distortions. Quasi-static aeroelastic methods based on lifting line theory were appropriate for flexible airplanes of the Boeing B-47 and Douglas DC-8 generation, subsonic airplanes with long, narrow wings. Proper quasi-static aeroelastic analysis of the lower aspect ratio, complex wing planforms of the Northrop B-2 stealth bomber and supersonic-cruise transport airplanes, requires panel methods.
NASTRAN is a widely used finite-element structural analysis computer program. The MacNeal-Schwendler Corporation’s proprietary version, called MSC/NASTRAN, adds
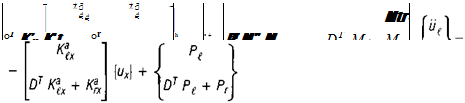 |
In this equation:
u = displacement vectors or column matrices К — structural stiffness matrices M = structural mass matrices P = aerodynamic force matrices D = a rigid body mode matrix
Figure 19.9 One form of the NASTRAN quasistatic aeroelastic matrix equations. Additional manipulations are needed to arrive at the unrestrained aeroelastic stability and control derivatives. (From Rodden and Johnson, eds., MSC/NASTRAN Aeroelastic Analysis User’s Guide, 1994)
aerodynamic finite-element models to the existing structural models with splining or interpolation techniques to connect the two. This version can perform quasi-static aeroelastic analysis (Figure 19.9). This accomplishment is credited to a number of people, including Drs. Richard H. MacNeal and William P Rodden, and E. Dean Bellinger, Robert L. Harder, and Donald M. McLean.