Improved Piston Theory: M2s2 = O( 1)

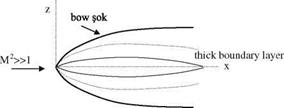
The leading edge shock of a thin airfoil is not weak even if it is attached. Therefore, it creates non negligible turning in the flow field. When this turning angle is multiplied with free stream Mach number, the product is usually in the order of unity, i. e., Мв * 1, similarly Ms * 1. For these cases we need a valid theory especially for the lifting surfaces in hypersonic aerodynamics, Fig. 7.7.

The development of the pertinent theory is based on the order of magnitude analysis performed on the unsteady Euler equations in terms of the Hayes’ simi­larity parameter. In this way we can obtain an expression for the unsteady lifting pressure for thin airfoils in hypersonic flow (Van Dyke 1954). Using the Van Dyke’s small perturbation theory it is possible to obtain the lifting pressure for arbitrary motion of an airfoil at high Mach numbers with small turning angles with utilization of shock formulae (Pierce 1978).

Let zv (x, t) = zo (x) f(t) be the equation of the chord motion of the profile shown in Fig. 7.7. The equation of the upper surface for small angle of attack a becomes

z — [z-(x) — ax + zv(x, t)] = 0 (7.28)

If the turning angle at the leading edge is hH, then for the upper and lower surfaces

= – —a and hi = l +a, (7.29)

dx x=0 dx x=0

respectively. The ratio between the value of the pressure right after the leading edge shock and the free stream pressure in terms of the shock angle b reads as (Liepmann and Roshko),

— = — [(bM)2 — 1] + 1 (7.30)

Pi c + 1

Here, b is the shock angle created by the leading edge turning angle dH and d(x) is the inclination of the surface given as

Now, we can enumerate the application areas of Eq. 7.34 as follows (Pierce

1978) .

1. As long as the angle of attack is small, the hypersonic similarity parameter satisfies the criterion and Eq. 7.34 is applicable for the flow past profiles with sharp trailing edges.

2. The Prandtl-Meyer expansion formula is used to obtain Eq. 7.34 for hypersonic flows with small turning angles. If the turning angle increases, the error also increases, therefore, it has to be corrected by exact shock formulae.

3. Equation 7.34 can be used for finite wings with the concept of strip theory. However, the strip theory is not valid at the sharp wing tips, and it is not capable of modeling the low aspect ratio wings with high sweep angle.

4. Profiles with round leading edges have detached shocks which makes the application of piston theory and the Prandtl-Meyer theory non valid. Therefore, we need to use some other formula for profiles with blunt noses.

The next step is studying the effect of the detached shock created by the round leading edge and the hypersonic flow field after the shock to numerically evaluate the surface pressure of some special geometry.