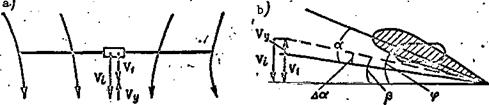Main Rotor Thrust in Vertical Climb and Vertical Descent

Main rotor thrust in vertical climb. It was established above that the thrust of the ideal main rotor in the hovering regime is defined by the formulas

The first formula is of a general nature and is applicable for all axial – flow regime cases. The second is applicable only for determining the thrust in the hovering regime.

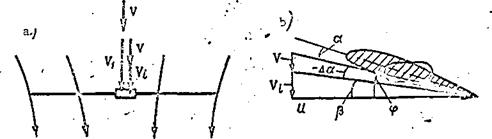
During vertical climb, the magnitude of the air mass flowrate m^ through the swept area changes. This is seen from the schematic of main rotor motion during vertical climb (Figure 23a). The rotor travels upward with the velocity V. We can say that an undisturbed flow caused by this motion approaches the rotor (principle of reversibility of motion). In the plane of rotor rotation, the flow velocity V^ will be

If the air mass flowrate is defined as m^ = pFV^, then the thrust is

Figure 23. Operation of main rotor in vertical climb.

defined as T = m„V, or T = pFV, V, , and since the equality V, = 2V. is also S dw 1 dw dw x

valid for vertical climb, the thrust will be

T = ^FV1V^-2oFViVl.

Comparing the main rotor thrust T = 2pFV^2 in the hovering regime, and

the thrust T = 2pFVjV^ in the vertical climb regime, we can say that the

thrust in the climbing regime is higher than that in the hovering regime, since

V, > V.. But this conclusion would be valid only if the induced velocity V. lx x

did not change with change of the rotor motion velocity. In actuality, the induced velocity decreases with increase of the translational velocity, which leads to reduction of the main rotor thrust.

This means that the main rotor must develop more thrust during vertical climb than the weight of the helicopter. The dependence of the main rotor thrust on speed can also be explained from the viewpoint of blade element theory. In helicopter hovering, the blade element angle of attack depends on the pitch and the induced flow velocity (Figure 15b).

With increase of the climb velocity, the angle of attack of the main
rotor blade element decreases, and therefore the main rotor thrust coefficient

Figure 24. Operation of main rotor in vertical descent.

decreases, which in turn leads to reduction of the main rotor thrust, since T = CTF (0)#)2 (Figure 23b) .

Main rotor thrust in vertical descent. During vertical descent (Figure 24a), the undisturbed flow approaches the main rotor from below with the velocity V ; therefore, the flow velocity in the plane of rotation of

the main rotor is V, = V. – V, i. e., it will be less than during hovering.

1 і у

Main rotor thrust in vertical descent is defined by the same formula as for vertical climb T ~Cj. F-%jrU* or T = 2pFV V

The main rotor blade element angle of attack is increased during vertical /32 descent by the amount Act as a result of the vertical descent velocity, which leads to increase of the coefficient and of the main rotor thrust (Figure 24b). Two flows are encountered below the rotor: the induced flow,

accelerated by the rotor, and the undisturbed flow created by descent of the

helicopter. Meeting of these two flows leads to the onset of instability of

the vortices, buffeting of the main rotor, and deterioration of control.