Our heavyweight helicopter equal in the world does not have
In Rostov started production of the most load-lifting rotary-wing car The Russian holding «Helicopt[...]
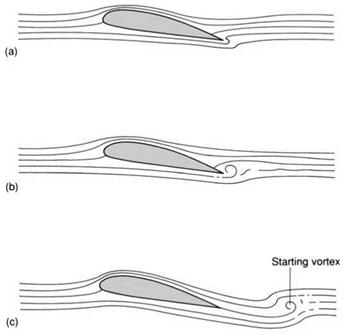
The generation of lift and the formation of the starting vortex
Figure 3.5 shows what happens when an aerofoil is rapidly accelerated from rest. At first, when virtually no lift is generated, the streamlines show an almost anti-symmetrical pattern, with a rear dividing position situated on the upper surface near the trailing edge. This pattern is similar to that given by earlier versions of the classical theory, where no lift was predicted (see Fig. 1.6(b)).
As the flow speed increases, the boundary layer starts to separate at the trailing edge, due to the adverse pressure gradient, and a vortex begins to form, as shown in Fig. 3.5(b).
The vortex grows, moving rearwards, until it eventually leaves the surface and proceeds downstream, as in Fig. 3.5(c). This detached vortex is the starting vortex that we described in Chapter 2. We can see that it is the production of this starting vortex that destroys the anti-symmetry of the flow, resulting in differences in pressure and speed between the upper and lower surfaces. Thus, it is viscosity, working through the mechanism of boundary layer separation and starting vortex formation, that is ultimately responsible for the generation of lift.
The upper and lower surface flows rejoin at the trailing edge with no abrupt change of direction; the Kutta condition mentioned in Chapter 1. The upper and lower surface boundary layers join to form a wake of air moving more slowly than the surrounding air stream.
|
Fig. 3.5 The formation of the starting vortex |
|
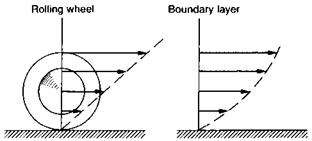
Fig. 3.6 The velocity variation in the boundary layer is rather like that in a wheel rolling along a surface, and may similarly be thought of as being a combination of rotational and translational movement |
In Chapter 1 we showed how the difference in the speeds above and below the wing could be represented as being equivalent to superimposing a circulating vortex type of flow on the main stream. By similar reasoning, we can say that, since the flow speed in the boundary layer is faster at the outside than at the surface, it too can be represented by a combination of rotation and translation, as illustrated in Fig. 3.6. Once again, it should be noted that no air particle actually goes round in circles. The flow in the boundary layer merely
has rotational tendency superimposed on its translational motion. However, if a speck of dust enters the boundary layer, it will rotate as it moves along.
