The Transfer Function Model for Unsteady Flow
Aerodynamicists familiar with the classical Bryan formulation of the perturbation equations of airplane motion expect to find aerodynamic forces and moments expanded in Taylor series. As an example, the yawing moment coefficient Cn is expanded as Cn = Cnp x в + Cnp x pb/2V + Cnr x rb/2V + Cns x 8 + ■ ■ ■. The series uses the first derivative only of the function (Cn) with respect to the independent variables, which are the vehicle’s state variables в, p, r, 8, etc. With this background, it is natural to treat unsteady flow effects by adding higher derivative terms to the expansion, such as Cn в x в.
Although mathematically sound, this approach has a serious flaw (Greenwell, 1998). Numerical values of higher order derivatives such as Cnp can be correct at only one oscillation frequency. Numerical values obtained in oscillating wind-tunnel rigs are correct at the frequency tested, but are in general invalid for the free or controlled angular motions of an airplane.
The solution of this problem is readily apparent to engineers trained in servomechanism theory. That is, treat aerodynamic force and moment as the result of dynamic processes much as hydraulic actuators and electrical networks are treated. The transfer function concept shown in Figure 10.2 is ideal for this application. Other modeling methods, such as Fourier function analysis, can produce equally valid results, but as Greenwell points out, the transfer function approach has the great advantage of being easily integrated into flight simulation computer codes (Abzug, 1997). Greenwell further proposes parallel transfer functions for applications at angles of attack that lead to separated flows and vortex bursting, each with its characteristic model. Transfer functions are not limited to first-order lag forms, but these have dominated the field so far. A first-order lag form adds one additional state to a state space aerodynamic model, as in the Goman and Khrabov example of Figure 10.11.
|
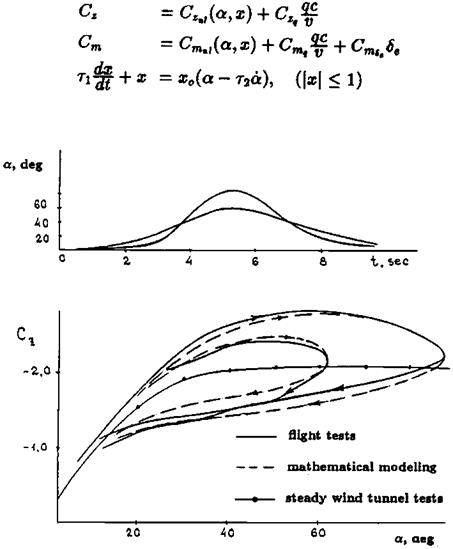
Figure 10.11 Time-dependent mathematical model for aerodynamic force and moments proposed by M. G. Goman and A. N. Khrabov for the fully stalled regime encountered in supermaneuvers. Below, lift coefficient variation with angle of attack, using this model. (From Zgainov, AIAA Paper 93-4737, 1993) |
The transfer function concept applied to modeling unsteady aerodynamics in simulations is typical of many developments in that it is difficult to establish priority. Greenwell credits Dr. Bernard Etkin as the originator of the concept, with his publication of a 1956 University of Toronto paper (UTIA Report 42). Early work on the concept also was done by Kenneth Rogers, Thomas Burkhart, J. Roy Richardson, Moti Karpel, William P Rodden, and R. Vepa.
A 100-state aeroservoelastic model ofthe GrummanX-29A forward-swept wing research airplane uses the transfer function model for unsteady aerodynamics. The transfer function model was also used with success at the DLR to model lift hysteresis at the stall for the Fairchild/Dornier Do 328 (Fischenberg, 1999) (see Chapter 14, Sec. 8.4).
10.3 The Inverse Problem
A requisite for linear analysis is a reference motion about which small perturbations occur. Generating reference motions in the case of fighter airplane supermaneuvers can be a particular problem. A planar reference maneuver, such as a Cobra-type snapup, may be generated with no particular difficulty from flight path equations (short-period dynamics suppressed) for a specific airplane. One would apply full nose-up aerodynamic or thruster control until the desired peak attitude or angle of attack is reached, followed by full nose- down control. This open-loop maneuver would yield a time history of airspeed, attitude, and angle of attack from which operating points could be selected for small-perturbation stability analysis.
Difficulties can be expected only if a maneuver path in inertial space is specified, rather than an open-loop time sequence of control or thruster angles. In that case, an inverse solution is required to determine the airplane’s velocity along the path and to be sure that the maneuver is possible in the sense that control limits are not exceeded. Again, specifying a planar reference trajectory for inverse solution presents no difficulty. The case is different for nonplanar maneuvers in that the geometry could become complex.
In principle, spatial sequences of six of the normal airframe states, the three position coordinates of the center of body axes and the three Euler angles, can define any airplane maneuver. A natural path set of coordinates has been proposed instead, particularly for nonplanar maneuvers (Myers, McRuer, and Johnston, 1987). The method is illustrated with the familiar yo-yo tactical maneuver. Natural path coordinates – tangent, normal, and binormal – are a familiar concept in classical mechanics.