Thrust and Power Required for Helicopter Descent

The thrust developed by the main rotor during flight along an inclined

trajectory must provide the necessary magnitudes of the lift force Y and its

component P parallel to the motion trajectory. In accordance with the dia – X

gram of the forces acting on the helicopter, the thrust force f = ]/"У2 – j – p*..

From (33) we find Y = G = G cos 0, and from (34) +P = G~ – X = G sin 0 –

1 – x 2 par

– X, then par

Since in most cases the descent angle 0 is small (less than 10°) cos 0^1. This means that the first term of the radicand in (35) is close to one and the second term is close to zero. Hence, we can conclude that the thrust required for helicopter descent along an inclined trajectory will be practically equal to the helicopter weight. For large descent angles, when the angle 0 approaches

90°, the first term of the radicand of (35) approaches zero, and the difference

о»

G sin 0 – X r approaches in magnitude the weight, i. e., T ^ G.

We came to the same conclusion in studying helicopter vertical descent. Comparing the thrust force required in the different helicopter flight regimes, we can say that the thrust required for flight in any regime is practically equal to the helicopter weight.

The power required for descent along an inclined trajectory consists of three parts: the motion power N , the power required to create the lift

force or inductive power N^, and the power required to overcome the profile drag If during descent the velocity and rpm are the same as in horizontal

flight, the profile power is the same in both cases. The induced power is found from the formula = YV^ = G cos 0 V^, and for descent angles up to 10° is practically equal to the induced power for horizontal flight, since in this

<Vi

case cos 9 ^ 1.

The motion power for descent along an inclined trajectory is found from the formula

N „ = P V.
mot x

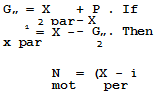par

The parasite drag forces are practically the same for descent and horizontal flight (for the same speed). Therefore

X V = N. par mot.

We denote G^V = ДЫ; then

i. e., the power required for descent is less than the power required for horizontal flight. Comparing (28), (32), (37), we can say that the most power is required for climbing flight and the least is required for descending flight The speed dependence of the power required for different flight regimes can be shown graphically with the aid of the Zhukovskiy grid, or by curves of the power required for various flight regimes, which are plotted for a given altitude (Figure 71a).