Derivation of the structural response

In general, the transverse vibration of a flexible thin shell structure is governed by three inhomogeneous equations of motion, which can be written in the following form (Soedel 1993; Markus 1998):

L1(u, v,w, xs, t) – ms d2u(xs, t)/dt2 = – fz (xs, t)

L2(u, v, w, xs, t) – ms d 2v(xs, t)/5t2 =-fg (xs, t) (23a-c)

L3(u, v, w, xs, t) – ms d2 w(xs, t)/5t2 =-fr (xs, t) .

Here Lt(u, v, w, xs, t) are differential operators for the theory used to describe the equations of motion of the shell structure. Also u, v, w and fz, fe, fr are the displacements and forces per unit surface along the three axis used to describe the geometry of the shell (in this section, the notation for cylindrical coordinates is used). Finally ms is the mass per unit surface of the shell structure and the vector xs defines a generic point on the shell structure. Normally, for thin shell structures, the response is derived from the so-called classical theory of thin shells established by Love. This theory assumes the shell is thin compared to the radii of curvature and the deflections are reasonably small. Also it neglects the effects produced by shear deflections and rotary inertia. After Love’s work, several refinement theories have been proposed for specific classes of shells, which are discussed in specialised texts such as, for example, those in references
(Graff 1975; Cremer et al. 1988; Leissa 1993; Soedel 1993; Markus 1998; Reddy 2006). Assuming time-harmonic motion, Eqs. (23a-c) become

L1(u, v, w, xs,®) + ®2msu(xs,®) = – fz(xs,®)

L2(u, v, w, xs,®) + ®2msv(xs,®) = – fe(xs,®) (24a-c)

L3(u, v, w, xs,®) + a1 msw(xs, m) = – fr (xs,®) ,

where, in this case, u, v,w and fz, fg, fr are the frequency dependent complex

amplitudes of the co-respective time-domain functions assuming the time – harmonic dependence is expressed in the complex exponential form exp( jat). Also in this case, for brevity, the frequency dependence of the complex amplitudes will not be displayed in the remaining part of the formulation. In general, for curved shell structures, the three equations of motion are coupled (Soedel 1993; Markus 1998).

The solution of Eqs. (24a-c) can be expressed with the following set of three series expansions in the m = 1, 2, … structural natural modes of the shell, (Morse and Ingard 1968):

u(xs) = Z^,rn (xs)bz, m

v(xs) = Z^,m (xs)bVm (25a-c)

m=1

w(xs) = Z^,m (xs)br, m ■ m=1

Here Ф^Фе^Фг,*, and bzm, bSm, brm are the components of the m-th natural mode

and the co-respective complex modal responses along the axis used to describe the geometry of the shell (the combination of the three functions ^zm,^,m,^.m

constitute a natural mode).

Considering the model problem studied in this chapter, the free vibration of in vacuo cylindrical structures is characterised by three families of natural modes: the axial modes that are dominated by longitudinal vibrations, the torsional modes that are controlled by circumferential vibrations and the flexural modes that are governed by radial vibrations (Junger and Feit 1986). Thus for each mode number there are three natural frequencies relative to the axial, circumferential and radial natural modes. Usually the lowest one corresponds to the predominantly radial (i. e. flexural) natural mode (Markus 1998). Moreover, for circular cylinders, these mode shapes show a periodicity in circumferential direction. Thus, in order to represent a generic vibration field, two mode components oriented orthogonally in circumferential direction must be used in

Eqs. (25a-c). Normally a symmetric cosine and an anti-symmetric sine function are employed. A comprehensive account on the derivation of natural frequencies and natural modes of thin cylinders can be found in (Junger and Feit 1986; Soedel 1993; Markus 1998). For the specific structural-acoustic problem considered in this section, the coupling of the interior/exterior sound and TBL pressure fields with the structure occurs predominantly via radial, i. e. flexural, response of the structure. Thus, the formulation for the structural vibration can be simplified by neglecting the axial and torsional natural modes and using simplified expressions for the flexural natural frequencies as described by (Junger and Feit 1986; Soedel 1993). For instance, for the simply supported circular cylindrical shell model problem of Figure 1, the derivation of the response in radial direction can be expressed with the following series expansion (Soedel 1993):

w(xs) = ZCrn Os)b“

where, for each mode index m, the summation is carried out considering symmetric (a = s) and antisymmetric (a = a) radial mode shapes. Also the natural frequencies and co-respective symmetric and anti-symmetric radial mode shapes are given by (Soedel 1993):

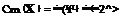(27)

(28a, b)

where ps, Es, vs are respectively the mass density, Young’s modulus of elasticity and Poisson ratio of the material and R, L, h are respectively the radius, length and thickness of the cylinder. These equations are given in cylindrical coordinates z,0,r so that m1 = 1, 2, 3, … and m2 = 0, 1, 2, … are the

modal indices in axial and circumferential directions for the m-th structural natural mode. The first term under the square root of Eq. (27) is controlled by the membrane stiffness of the cylinder and tends to reduce to zero for increasing circumferential mode orders m,. Alternatively, the second term under the square

root of Eq. (27) is controlled by the bending stiffness of the cylinder and becomes progressively important for increasing circumferential mode orders m2.

The unknown complex modal amplitudes brs, m and bra, m can be derived by substituting Eq. (26) into Eq. (24c). The resulting equation is then multiplied by

a m – th mode and integrated over the surface of the cylinder so that, using the orthormality property of the natural modes and assuming the natural modes are mass normalised, the following set of uncoupled ordinary equations is obtained:

M(<m -®2 K“m = f“m m = 1, 2 -, ® « = S a, (29)

where M is the mass of the shell. Thus for each mode order m, two equations are defined with respect to the symmetric and anti-symmetric complex modal amplitudes bsrm and barm. Also, f “m is the m-th modal excitation term for either

the symmetric or anti-symmetric mode shape, which, for the specific case of radial excitation only, is given by (Soedel 1993):

f :m Cm(Ofr(K)dSb. (30)

Thus, according to Eqs. (26) and (29), the velocity response in radial direction of the cylinder can be expressed with the following modal summation

In general, analytical expressions for the natural frequencies and natural modes can be derived only for regular shapes such as, for example, circular cylinder closed shells or flat and curved shells panels. When the problem at hand involves complex wall structures, the Finite Element Method (FEM) numerical technique is normally employed (Fahy and Gardonio 2007).

The energy dissipation in the structure is normally modelled with a hysteretic damping model (Cremer et al. 1988), which, leads to a complex stiffness in modal coordinates so that the Eq. (31) becomes

where r/ is the loss factor. The interaction between a structure and the surrounding fluid also produce energy dissipation. For slender structures this effect is normally modelled in terms of viscous damping without carrying out a coupled structural-acoustic analysis. In particular, the so called Rayleigh damping model is used, so that the damping can be modelled in terms of viscous damping coefficients in modal coordinates. Alternatively, for structures with extended surfaces such as the cylinder problem considered in this section, the true dissipation effects are modelled with a coupled structural-acoustic analysis, which is presented in the forthcoming section.