Kinetic heating

We all know that friction increases temperature, an example of deterioration of energy from the highest to the lowest form (from mechanical energy to thermal energy), a natural process – and skin friction in the flow of fluids is no exception. We all know, too, that an increase of pressure, as in a pump, raises temperature – and the stagnation pressure on the nose of a body or wing is no exception. So when an aeroplane moves through the air it gets hot; some parts more than others, some owing to the temperature increase created by skin fric­tion, some owing to that created by pressure.

When, then, do we first come up against this? The answer is – when we first fly! But it isn’t serious? No – like many other things, it isn’t serious at low speeds. It has been said that aeroplanes made of wax melt at 300 to 400 knots, those made of aluminium at 1600 to 1800, those of stainless steel at 2300 to 2400 knots. Aeroplanes are not made of wax (wind tunnel models sometimes have been), but some are made of aluminium alloy, and some of stainless steel, and of other metals (such as titanium) and their alloys, and just because of this very problem. Nor can we afford to go anywhere near the melting point; metals are weakened long before that – and what about the passengers, and crew, and freight?

Bullets and shells certainly travel at speeds where heating is significant, but within limits it doesn’t matter whether bullets and shells get hot or not. Also their flight is not usually of very long duration, and it takes time for the surface to heat up. But meteors and satellites re-enter the atmosphere without any means of braking – and we know what happens to them, they get frizzled up. It is true that most manned space-craft have survived re-entry and more will be said about that in the next chapter. Let us at least consider what we can do to reduce heating effects.

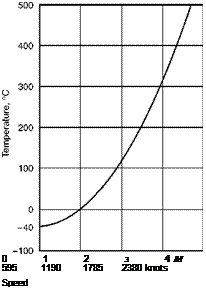
A very simple formula (V/100)2, where V is the speed in knots, gives a very fair approximation to the temperature rise in degrees Celsius. So what is merely a rise of 1°C at 100 knots, or 4°C at 200 knots, becomes 36°C at 600, 100°C at 1000, and 400°C at 2000 knots. That is how we discovered that the aeroplane made of wax would melt! Figure 12.26 shows rather more accu­rately local surface temperatures that may be reached under certain conditions at Mach numbers up to 4; these have been calculated from the formula t/T = (1 + M2/5) where t is the stagnation temperature, i. e. the temperature of air moving at a Mach Number of M being brought to rest, and T is the local tem­perature of the air; the figures relate to 8500 m where the local temperature is
—40°C. The temperatures shown in the graph apply to a laminar boundary layer; the temperatures are rather higher for a turbulent boundary layer. Moreover, at Mach Numbers above 2 these surface temperatures may be reached in a matter of seconds, and certainly within a minute or two, unless there is some method of insulation.

Many devices have been tried, and no doubt many more will be tried, in an effort to counter this heating problem. These devices may be classified under the following headings –

(a) To insulate the structure from the heat.

(b) To use materials which can stand the high temperatures without serious loss of strength.

(c) To encourage radiation from the surfaces and so reduce the temperatures.

(d) To circulate a cooling fluid below the surface.

(e) Refrigeration by any of the normal methods.

As regards materials for the aircraft structure light alloys are suitable for Mach Numbers up to 2, or even higher for short periods. Between М2 and M4 titanium alloy may be the answer, but above 3 or 3.5 stainless steel is probably better as being more readily available.

Fig 12.26 How the surface temperature rises with the Mach Number The graph relates to a height of 28 000 ft (8500 m) where the local temperature of the surrounding air is 40°C.

It must be remembered that the crew, the equipment and the fuel must be protected as well as the structure itself, so there is no point in using materials which will stand the high temperatures, unless there is also refrigeration to keep the interior of the aircraft cool.

Perhaps the most ingenious idea is to apply the heat to a suitable working fluid (hydrogen has been suggested), and to eject the expanding fluid through a suitable nozzle, and so propel the aircraft! Ingenious and fascinating – drag produces heat, heat produces thrust to help overcome the drag. In principle it is not impossible.

An interesting aspect of surface heating is the effect of shape. It is the speed of flow adjacent to the boundary layer which is the deciding factor in the tem­perature rise – and to some extent, of course, the nature and thickness of the boundary layer itself – and the speed of flow depends on the shape of the body. But there is more in it than that. A rise in temperature is created owing to skin friction, and owing to the stagnation pressure, but it is also created by shock waves, and whereas the main effect of skin friction in the boundary layer is to raise the temperature of the surface, the main effect of the shock waves is to raise the temperature of the air – and that doesn’t matter very much. So from the point of view of keeping down surface temperatures it is better to have wave drag than boundary layer drag. This conclusion isn’t very helpful with regard to aircraft in which we try to reduce every kind of drag to a minimum, but it is a most important consideration in designing bodies for re-entry to the atmosphere from space, bodies in which we want drag, but we don’t want heating of the surfaces.

Another influence of the heating problem on shape is in the avoidance of sharp edges, which might seem desirable from the flow point of view, but which would be particularly susceptible to local temperature rise and conse­quent weakening of the material.

Kinetic heating is already a limiting factor in the speed of certain types of aircraft, and it provides a very formidable problem in regard to the re-entry into the atmosphere of spacecraft and even of long-range missiles such as will be considered in the next chapter.