AXIAL FLIGHT: VERTICAL DESCENTS AND THE VORTEX-RING STATE

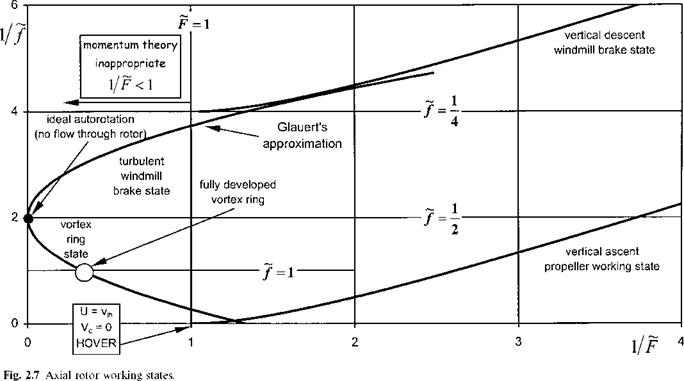
Momentum theory and simple blade element theory are based on constant energy flow above and below the rotor. They both also assume that the slipstream has a finite velocity either side of the rotor. When these conditions are not satisfied these theories can no longer be used to make theoretically accurate predictions. Provided the rotor ascends, continuous flow through the rotor exists and use of the momentum theory is valid. The hover therefore represents the lowest vertical climb speed for which momentum theory is sound. Consider a vertical descent at low rates of descent: the rotor is now working to impart a downwards velocity on the fluid whilst the general flow along the streamtube is upwards. Clearly smooth flow is not possible in this situation and thus a vortex will form in the region of the rotor. A limiting condition arises when the rate of descent matches the induced velocity necessary to generate the thrust. Here the flow stagnates in the disk and fully developed vortex flow has been established. Such a situation leads to a rapid descent with uncontrolled pitching and rolling motion being caused by a violent flapping motion of the rotor blades. At rates of descent above this limiting value a steady smooth flow upward through the rotor is established and the momentum theory can be applied. It is possible to determine the value of VD ( — Vc) equating to fully developed vortex ring flight. It is often surmised that provided VD exceeds the induced velocity required for hovering flight then the rotor is extracting sufficient energy as it descends through the atmosphere to support the weight of the helicopter. Thus momentum theory:

• applies in vertical ascents and in the hover (when Vc p 0);

• is invalid in the vortex-ring and turbulent windmill-brake states (when 0 < VD < 2vih);

• applies in the windmill-brake state (when VD > 2vih).

The variation of induced velocity through all phases of axial flight can be found using a combination of momentum theory for the vertical ascent and windmill-brake states and empirical relationships for the vortex-ring and turbulent windmill-brake states. An expression developed by Glauert [2.3] has found favour:

3f 2

(1 — f) 2

where:

2

U = Vc±v,

Plotting the variation of 1/f with 1/F, see Fig. 2.7, is a convenient method of portraying the different flow states. As has already been mentioned momentum theory cannot be applied for rates of descents less than twice the induced velocity. It can be shown that this condition equates to a value off < 0.25. The hover condition relates to a value of F equal to zero. These two conditions form boundaries inside which non-momentum flow takes place.