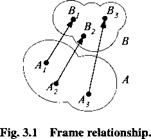
Frames

Recall the definition from Chapter 2: A frame is an unbounded continuous set of points over the Euclidean three-space with invariant distances and which possesses, as a subset, at least three noncollinear points. The inertial frame is such an unbounded set of points. We will give it a precise definition shortly. The Earth, although bounded, has also a frame associated with it. Theoretically, the Earth frame extends beyond the confines of the geoid, but when we refer to the points of the Earth’s frame, we remain on the Earth. A similar approach is taken with the body frame. Strictly speaking, the body must be rigid to be modeled by a frame. For elastic modeling it is common practice to divide the body into finite, rigid elements, each of which is represented by a frame.

We must be able to identify at least three noncollinear points of a frame. Oth­erwise, the frame may not occupy the three-dimensional manifold of space. In particular, we may pick the three points such that, if connected with a fourth base point, they establish a triad that defines the position of the frame completely.