Froude theory

The Froude theory postulates that the flow above and below a climbing rotor can be considered as constant energy with energy being imparted only by the actuator disk. This energy is added to the airflow in the form of an increase in static pressure. The theory then draws some conclusions about the streamtube that the disk influences. Far above the rotor, the velocity of flow in the streamtube is equal to the freestream that is dependent on the rate of climb of the rotor itself. As the rotor draws air through its disk the velocity just above the disk is greater than the freestream and as a consequence of the continuity equation the streamtube will have contracted; also by virtue of Bernouilli’s relationship the pressure just above the rotor will be less than ambient. Immediately below the disk the pressure will be greater than ambient because of the energy added by the rotor however, the velocity and streamtube area will be the same as just above the rotor. Far below the disk in what is termed the ultimate wake, the flow will achieve a pressure equal to ambient but the velocity will exceed the freestream again because of the energy imparted by the rotor. The continuity equation and Bernoulli relationship require that the cross-sectional area of the ultimate wake be less than the disk area, see Fig. 2.1. Adoption of the concept of an actuator disk leads to a very simple relationship for the thrust developed by a rotor:

T = A(P2 — p)
where A is the disk area.

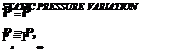The pressure difference (P2 — Pr) generated by the disk can be related to the vertical velocity by considering the changes in pressure and velocity occurring in the streamtube. Consider a helicopter climbing vertically at speed Vc and assume that the

Vr + kv,

Fig. 2.1 Flow through the actuator disk.

acceleration of flow caused by the action of the rotor results in an increase in the flow velocity of vi (Fig. 2.1). Likewise, assume that the continued acceleration of flow below the rotor leads to a total velocity rise of kvi at the ultimate wake. Now Bernoulli states that:

P„ + 2 pvc2 = p + 2 P(VC + vi )2 P2+2 p( Vc + vi )2 = Pm+2 p( Vc + kvi )2

Thus:

T = A(P2 – Pt) = 2 pA(2VC + kvi)kvi

This relationship requires a value for k before it can be used to estimate the thrust required for a given rate of climb. If the momentum change caused by the disk is considered, an alternative expression for thrust can be developed. Recalling that force is equal to rate of change of momentum or massflow multiplied by a change in velocity gives [2.2]:

T = pA(VC + vi )kvi

Hence k= 2 and:

T = 2pA(VC + vi )vi (2.1)

This fundamental equation predicts that the induced velocity at the rotor disk is equal to half the total increase in flow velocity required to match the thrust requirement of the rotor. The maximum increase in velocity occurs at the ultimate wake, which is usually taken as one rotor diameter downstream of the disk. This momentum disk model can be applied to any working state of the rotor in which a continuous streamtube is formed.